2 Answers
### 1. **Oscillation Principle**
The core principle of a sinusoidal oscillator is to produce a continuous waveform that alternates smoothly in a periodic manner. This is achieved by creating a feedback loop that reinforces the signal at its fundamental frequency, ensuring a stable oscillation.
### 2. **Positive Feedback**
A sinusoidal oscillator relies on positive feedback to maintain oscillations. Positive feedback means that a portion of the output signal is fed back into the input of the circuit in such a way that it enhances or sustains the oscillation. In practical terms, this feedback must be carefully controlled to ensure that the amplitude and frequency of the output waveform are stable.
### 3. **Phase Shift**
The oscillator circuit must incorporate a phase shift network, which introduces a phase shift of 180 degrees. This is crucial because, to sustain oscillation, the feedback loop needs to ensure that the total phase shift around the loop is 360 degrees (or 0 degrees, which is effectively the same due to periodicity). Typically, this is achieved using combinations of inductors, capacitors, and resistors.
### 4. **Frequency Determination**
The frequency of oscillation is determined by the components in the phase shift network. For instance:
- **In a Wien Bridge Oscillator:** The frequency is set by a resistor-capacitor (RC) network. The frequency \( f \) is given by \( f = \frac{1}{2 \pi R C \sqrt{2}} \), where \( R \) and \( C \) are the values of the resistors and capacitors in the circuit.
- **In a Colpitts Oscillator:** The frequency is determined by the capacitors and the inductor in the circuit. The formula involves the capacitances and the inductance, such as \( f = \frac{1}{2 \pi \sqrt{L (C1 + C2) / (C1 C2)}} \), where \( L \) is the inductance and \( C1 \) and \( C2 \) are the capacitors.
### 5. **Gain and Stability**
To achieve sustained oscillations, the circuit must have a gain equal to or greater than one. This ensures that the oscillations are neither damped nor grow uncontrollably. In many oscillator designs, an automatic gain control or a non-linear component (such as a light bulb or thermistor in the Wien Bridge Oscillator) helps stabilize the amplitude of the oscillation.
### 6. **Circuit Components**
- **Amplifier:** Provides the necessary gain and can be configured as a transistor, operational amplifier (op-amp), or a dedicated oscillator IC.
- **Frequency-Determining Network:** Consists of reactive components (inductors and capacitors) that set the oscillation frequency through their combined reactance.
- **Feedback Network:** Ensures that the output is fed back to the input with the correct phase shift to maintain oscillation.
### 7. **Non-Linearity**
Many sinusoidal oscillators use non-linear components to stabilize the amplitude. For example, the Wien Bridge Oscillator uses a non-linear resistance in the feedback loop to automatically adjust the gain, keeping the amplitude stable.
### Summary
In essence, a sinusoidal oscillator operates based on the principles of positive feedback, phase shifting, frequency determination through reactive components, and gain control. By carefully designing the feedback loop and phase shift network, the oscillator can produce a stable sinusoidal output that is used in various applications, including signal generation, clock pulses for digital circuits, and radio frequency (RF) transmissions.
### 1. **Positive Feedback**
- **Essential for Oscillation**: A sinusoidal oscillator relies on positive feedback, where a portion of the output signal is fed back to the input in phase with the original signal. This feedback amplifies the signal and sustains the oscillations.
- **Loop Gain**: The total loop gain (product of the amplifier gain and the feedback network gain) must be equal to or greater than 1 for sustained oscillations. If the loop gain is exactly 1, the oscillator will produce a stable, constant amplitude sinusoidal waveform.
### 2. **Frequency Determination**
- **Resonant Frequency**: The frequency of oscillation is primarily determined by the frequency-selective components in the feedback network, such as resistors, capacitors, and inductors. These components form a resonant circuit, which dictates the natural frequency of oscillation.
- **Oscillator Types**: Different types of sinusoidal oscillators (e.g., RC, LC, and crystal oscillators) use different frequency-determining elements:
- **RC Oscillators**: Use resistors and capacitors to set the oscillation frequency (e.g., Wien Bridge Oscillator).
- **LC Oscillators**: Use inductors and capacitors (e.g., Hartley and Colpitts Oscillators).
- **Crystal Oscillators**: Use a quartz crystal to achieve highly stable and precise frequencies.
### 3. **Barkhausen Criterion**
- **Oscillation Condition**: According to the Barkhausen criterion, for sustained oscillations:
1. The loop gain must be equal to 1 (|Aβ| = 1).
2. The total phase shift around the feedback loop must be 0° or an integer multiple of 360°.
- **Phase Shift Networks**: Oscillators include networks that provide the required phase shift so that the feedback signal reinforces the oscillations rather than dampening them.
### 4. **Amplification**
- **Amplifier Role**: The oscillator circuit typically includes an amplifier (such as a transistor or operational amplifier) to compensate for losses in the feedback network and maintain the oscillation amplitude.
- **Stabilization**: To prevent the amplitude from growing uncontrollably, some form of amplitude stabilization (such as automatic gain control) is often incorporated.
### 5. **Energy Exchange**
- **Tuning and Damping**: The oscillator operates by exchanging energy between the inductive and capacitive elements (in LC oscillators) or between the capacitive elements and resistive elements (in RC oscillators). Proper tuning ensures minimal damping, leading to a stable oscillatory output.
### 6. **Initial Conditions**
- **Startup Mechanism**: To initiate oscillation, some initial noise or disturbance in the circuit is usually sufficient. This small signal is amplified by the positive feedback until it reaches a steady-state amplitude.
### Summary
- **Positive Feedback**: Required for sustained oscillations.
- **Frequency Determination**: Set by the resonant frequency of the feedback network.
- **Barkhausen Criterion**: Ensures proper conditions for oscillation.
- **Amplification**: Maintains the oscillation amplitude.
- **Energy Exchange**: Between reactive components for stable oscillation.
- **Initial Conditions**: A small disturbance initiates oscillation.
Understanding these principles is crucial for designing and analyzing sinusoidal oscillators in various electronic systems.

Applied Physics

Signals and Systems

Digital Electronics
Basic Concepts
Basic Laws
Units

Ohmic Resistors
Capacitors and Inductors

RC Circuit
First-Order Circuits
Second-Order Circuits
Principles Of Circuit Analysis
Sinusoids and Phasors
AC Steady-State Analysis
Single Phase A.C. Circuits
Three-Phase Circuits
Resonance In Series And Parallel Circuits
Network Theorems
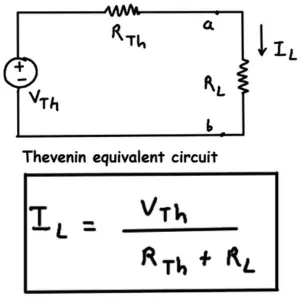
Thevenin's Theorem
Two-port Networks
Digital Electronics
Oscilloscope

Ohmmeter

Voltmeter
Ammeter

Induction Motor
Transformer
Operational Amplifiers
Components
Symbols
Formulas
EE Notes
EE Dictionary

MCQ Quiz

Interview Q&A
Power Electronics Book
Advanced Calculator

Basic Calculator
Simulator
Videos
Q&A

Capacitance Meter
Two Way Switch
Electrical Machines
Power Electronics

Electrical Drives & Their Control

Electrical Safety & Standards

Basics of Electronics Engineering
Electromagnetic Fields

Electrical Machines

More Items Coming Soon...