2 Answers
### Definition of Steady-State Analysis
Steady-state analysis involves examining the behavior of a system once it has reached a stable condition over time. In this state, the variables describing the system (such as voltage, current, pressure, and flow rates) do not change with time, or they fluctuate around a constant value. This contrasts with transient analysis, where the system is analyzed while it is still responding to changes, and the variables are time-dependent.
### Key Characteristics of Steady-State
1. **Time Independence**: In the steady state, the parameters of the system are constant (or periodic) over time. For example, in an electrical circuit, the voltages and currents remain constant once the system reaches steady-state.
2. **Equilibrium**: The forces, energy, and mass transfers within the system are balanced. For instance, in a mechanical system, the net forces acting on an object are zero.
3. **Periodic Behavior**: In some systems, particularly those subjected to periodic inputs (like AC circuits), the system may exhibit periodic steady-state behavior where the variables oscillate but with a constant frequency and amplitude.
### Applications of Steady-State Analysis
Steady-state analysis is widely used in:
1. **Electrical Engineering**:
- Analyzing circuits under sinusoidal steady-state conditions to find voltages and currents using techniques like phasor analysis or impedance methods.
- Designing and optimizing electrical systems, such as power distribution networks and amplifiers, ensuring they operate efficiently at steady-state.
2. **Mechanical Systems**:
- Evaluating the performance of mechanical systems such as engines or turbines when operating at a constant load.
- Ensuring that structural components can withstand steady-state loads without failing.
3. **Thermodynamics**:
- Analyzing heat exchangers, boilers, and refrigerators when operating at a constant temperature and pressure, ensuring efficient energy transfer.
4. **Fluid Dynamics**:
- Studying the flow of fluids in pipelines and systems when the flow rate remains constant, helping in designing efficient transportation systems.
### Techniques for Steady-State Analysis
- **Mathematical Modeling**: Developing differential equations or algebraic equations that describe the system's behavior under steady conditions. For example, in electrical circuits, Kirchhoff’s laws can be applied to find steady-state voltages and currents.
- **Simulation Tools**: Utilizing software tools like MATLAB, Simulink, or specialized circuit simulation software to model and analyze systems to predict steady-state behavior accurately.
- **Graphical Methods**: Techniques like Bode plots or Nyquist plots in control theory help visualize the steady-state response of systems, particularly in control and feedback applications.
### Example: Electrical Circuit Steady-State Analysis
Consider an RLC circuit (comprising a resistor, inductor, and capacitor) subjected to a sinusoidal voltage source. In steady-state analysis:
- We assume the circuit has been operating long enough that the initial transients (due to charging and discharging of capacitors or inductors) have died out.
- We can use phasor representation to express voltages and currents, leading to the calculation of impedance and phase angles.
In this case, the steady-state currents and voltages can be found using techniques such as:
- **Mesh or Nodal Analysis**: To set up the equations based on the circuit topology.
- **Impedance Analysis**: To convert the circuit components into their frequency domain representations.
### Conclusion
Steady-state analysis is a fundamental approach used to simplify complex systems by focusing on their behavior under stable conditions. Understanding this concept is essential for designing and optimizing systems in various engineering disciplines. By applying steady-state analysis, engineers can ensure systems operate efficiently, predict performance, and troubleshoot issues effectively.
Here’s a more detailed breakdown:
### Definition
**Steady-state** refers to a condition where the variables of a system (such as voltages, currents, temperatures, etc.) have stabilized and do not change over time. In electrical circuits, this usually means that any initial transients have settled, and the system operates in a stable manner.
### Key Aspects
1. **Time-Invariant Behavior**: In steady-state analysis, the assumption is that the system’s behavior does not change over time. This means that any initial transient effects or fluctuations have disappeared, and the system reaches a constant or periodic behavior.
2. **Sinusoidal Steady-State**: For AC circuits, steady-state analysis often involves analyzing the circuit’s response to sinusoidal inputs. In this case, the analysis focuses on how the circuit behaves with a constant sinusoidal source after all transient effects have settled.
3. **DC Steady-State**: For DC circuits, steady-state analysis involves examining the circuit’s behavior with constant DC sources, assuming that all transient effects from switching on or off the circuit have decayed.
### Techniques
1. **Phasor Analysis**: For AC circuits, steady-state analysis often uses phasor representation. Phasors simplify the analysis of sinusoidal voltages and currents by converting time-dependent sinusoidal functions into complex numbers (phasors). This makes it easier to apply Ohm’s Law and Kirchhoff's Laws in the frequency domain.
2. **Frequency Domain Analysis**: In steady-state analysis of AC circuits, components like resistors, inductors, and capacitors are analyzed in terms of their impedance (resistance in the frequency domain). This approach helps in solving circuits with sinusoidal sources efficiently.
3. **Laplace Transform**: In some cases, the Laplace transform is used to analyze systems in the frequency domain, and then the inverse Laplace transform is applied to determine the time-domain behavior. Steady-state analysis focuses on the behavior as \( t \to \infty \), ignoring transient effects.
### Applications
- **AC Circuits**: Steady-state analysis helps in determining the amplitude and phase of voltages and currents when subjected to sinusoidal sources.
- **Control Systems**: In control systems, steady-state analysis evaluates how well the system maintains a desired output in response to a constant input.
- **Signal Processing**: It is used to analyze how signals are filtered or modified by systems once transient responses have settled.
### Summary
Steady-state analysis is crucial for understanding the long-term behavior of systems once they have stabilized. In electrical engineering, it involves applying techniques like phasor analysis and impedance calculations to simplify and solve circuits under steady operating conditions.

Applied Physics

Signals and Systems

Digital Electronics
Basic Concepts
Basic Laws
Units

Ohmic Resistors
Capacitors and Inductors

RC Circuit
First-Order Circuits
Second-Order Circuits
Principles Of Circuit Analysis
Sinusoids and Phasors
AC Steady-State Analysis
Single Phase A.C. Circuits
Three-Phase Circuits
Resonance In Series And Parallel Circuits
Network Theorems
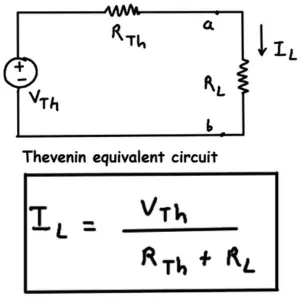
Thevenin's Theorem
Two-port Networks
Digital Electronics
Oscilloscope

Ohmmeter

Voltmeter
Ammeter

Induction Motor
Transformer
Operational Amplifiers
Components
Symbols
Formulas
EE Notes
EE Dictionary

MCQ Quiz

Interview Q&A
Power Electronics Book
Advanced Calculator

Basic Calculator
Simulator
Videos
Q&A

Capacitance Meter
Two Way Switch
Electrical Machines
Power Electronics

Electrical Drives & Their Control

Electrical Safety & Standards

Basics of Electronics Engineering
Electromagnetic Fields

Electrical Machines

More Items Coming Soon...