2 Answers
### Statement of Tellegen's Theorem
**Tellegen's Theorem** states that for any electrical network consisting of passive components (like resistors, inductors, and capacitors), the sum of the power across all branches of the network is zero at any instant of time. This can be expressed mathematically as:
\[
\sum_{k=1}^{N} v_k \cdot i_k = 0
\]
where:
- \(v_k\) is the voltage across the \(k^{th}\) branch,
- \(i_k\) is the current flowing through the \(k^{th}\) branch,
- \(N\) is the total number of branches in the network.
### Key Concepts
1. **Network**: An electrical network can consist of various components connected in different configurations, including resistors, capacitors, inductors, and independent or dependent sources.
2. **Branch**: A branch in a network refers to a single component connecting two nodes. Each branch has a voltage across it and a current through it.
3. **Power**: The product \(v_k \cdot i_k\) represents the instantaneous power associated with the \(k^{th}\) branch. Positive power indicates power delivered to the branch, while negative power indicates power absorbed from the branch.
### Implications of Tellegen's Theorem
1. **Conservation of Energy**: The theorem reflects the principle of conservation of energy. In a closed network, the total power supplied by sources equals the total power consumed by passive components, ensuring that energy is neither created nor destroyed.
2. **Applicability**: Tellegen’s theorem is applicable to a wide variety of circuits, including both linear and non-linear, as long as the components are passive. This includes circuits with resistive, capacitive, and inductive elements.
3. **Analysis**: The theorem provides a powerful tool for analyzing complex circuits, especially when combined with other techniques like Kirchhoff's laws or Thevenin's and Norton's theorems. It helps verify the consistency of circuit equations and the validity of power calculations.
### Sums Related to Tellegen's Theorem
While the primary equation given by Tellegen’s theorem is a single summation over the branches of the network, it can be broken down into more specific aspects or sums:
1. **Sum of Powers**: If we define the total power supplied by independent sources and the total power consumed by the passive components separately, we can express:
\[
P_{\text{sources}} = -P_{\text{passive}}
\]
This indicates that the sum of power delivered by sources equals the negative of the power absorbed by the components.
2. **Node Analysis**: By applying Tellegen's theorem across different nodes in a circuit, we can derive conditions for node voltages and currents, leading to a system of equations that can be solved to find unknown values.
3. **Component Analysis**: For each component, we can analyze the contributions of voltage and current to the total power, allowing for targeted assessments of performance and efficiency.
### Conclusion
Tellegen's theorem is an essential theorem in the field of electrical engineering and network analysis. It encapsulates critical aspects of power conservation and energy flow within electrical networks, making it a valuable principle for circuit designers and engineers. By applying the theorem, one can gain insights into the functionality of circuits, verify the correctness of network models, and ensure the efficient operation of electrical systems.
Understanding and utilizing Tellegen’s theorem is crucial for anyone involved in circuit design, analysis, or education, as it serves as a foundational principle that bridges various concepts in electrical engineering.
To express it more formally, Tellegen's Theorem can be stated as:
\[ \sum_{k=1}^{N} V_k \cdot I_k = 0 \]
where:
- \( V_k \) is the voltage across the \( k \)-th branch.
- \( I_k \) is the current through the \( k \)-th branch.
- \( N \) is the total number of branches in the network.
### Understanding Tellegen's Theorem
1. **Applicability**: The theorem applies to any linear network, whether it's electrical or any other type of network with linear components (like resistors, capacitors, inductors). It holds regardless of the specific values of the components or their arrangement.
2. **Power Consideration**: Tellegen’s theorem highlights that the total power supplied by the sources in the network equals the total power dissipated by the resistors. This is important for verifying that power is conserved in the network.
3. **Applications**: The theorem is often used in the analysis of complex networks and systems, as it provides a way to verify the correctness of circuit calculations and designs. It's particularly useful for checking the balance of power in circuits and understanding how energy is distributed.
### Example
Consider a simple circuit with a voltage source \( V \) and two resistors \( R_1 \) and \( R_2 \) in series. The current \( I \) flowing through the circuit can be computed as:
\[ I = \frac{V}{R_1 + R_2} \]
The power supplied by the voltage source is:
\[ P_{supply} = V \cdot I \]
The power dissipated by each resistor is:
\[ P_{R1} = I^2 \cdot R_1 \]
\[ P_{R2} = I^2 \cdot R_2 \]
According to Tellegen's Theorem:
\[ P_{supply} = P_{R1} + P_{R2} \]
So, if you calculate these values and find that they balance, it confirms that the theorem is holding true for your circuit.
In summary, Tellegen’s Theorem provides a powerful tool for analyzing and understanding electrical circuits, ensuring that power conservation principles are upheld.

Applied Physics

Signals and Systems

Digital Electronics
Basic Concepts
Basic Laws
Units

Ohmic Resistors
Capacitors and Inductors

RC Circuit
First-Order Circuits
Second-Order Circuits
Principles Of Circuit Analysis
Sinusoids and Phasors
AC Steady-State Analysis
Single Phase A.C. Circuits
Three-Phase Circuits
Resonance In Series And Parallel Circuits
Network Theorems
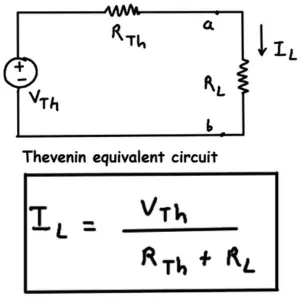
Thevenin's Theorem
Two-port Networks
Digital Electronics
Oscilloscope

Ohmmeter

Voltmeter
Ammeter

Induction Motor
Transformer
Operational Amplifiers
Components
Symbols
Formulas
EE Notes
EE Dictionary

MCQ Quiz

Interview Q&A
Power Electronics Book
Advanced Calculator

Basic Calculator
Simulator
Videos
Q&A

Capacitance Meter
Two Way Switch
Electrical Machines
Power Electronics

Electrical Drives & Their Control

Electrical Safety & Standards

Basics of Electronics Engineering
Electromagnetic Fields

Electrical Machines

More Items Coming Soon...