2 Answers
### 1. **Between Two Equal and Opposite Charges**
If you have two point charges of equal magnitude but opposite signs, the electric field can be zero at a point along the line connecting them. The electric field contributions from both charges cancel each other out at this specific point.
- **Example:** Imagine a positive charge \( +Q \) and a negative charge \( -Q \) placed a distance \( d \) apart. The electric field will be zero at a point on the line joining these charges where the distances from the point to each charge are equal.
### 2. **At Certain Points in a Uniformly Charged Ring or Disk**
In the case of a ring or disk with uniform charge distribution, there are points where the electric field cancels out.
- **Ring of Charge:** For a ring of charge, the electric field can be zero along the axis of the ring. Specifically, there are points along this axis where the contributions of the electric field from all parts of the ring cancel out.
- **Disk of Charge:** For a uniformly charged disk, the electric field can be zero along the axis perpendicular to the disk at certain points. The field strength varies with distance from the disk, and in some regions, it can reach zero.
### 3. **In Regions of Space Between Two Infinite Parallel Plates**
In the case of two infinitely large, parallel plates with equal and opposite charge densities, the electric field between the plates is constant and directed from the positive to the negative plate. However, outside these plates (beyond their edges), the electric field can be zero because the fields from the two plates effectively cancel each other out.
### 4. **At the Origin of a System with Symmetric Charge Distribution**
For a system with symmetric charge distribution, such as a uniformly charged sphere or a system with spherical symmetry, the electric field at the center of the distribution can be zero if the distribution is uniform and there are no other external fields influencing the region.
### 5. **In Certain Superposition Scenarios**
In general cases involving multiple charges, the electric field can be zero at a point where the vector sum of the electric fields due to all individual charges is zero. This is a result of the principle of superposition, which states that the total electric field at any point is the vector sum of the fields due to each charge.
### Summary
The key idea is that the electric field can be zero at points where the contributions from different charges or fields exactly cancel out each other. These points depend on the specific configuration of charges or field sources.
1. **In the Presence of Symmetrical Charge Distributions:**
- **Between Opposite Charges:** In a system with two point charges of equal magnitude but opposite sign, there exists a point along the line connecting the two charges where the electric field is zero. This point is equidistant from both charges.
- **In a Uniform Electric Field:** In a region where there is a uniform electric field, such as between the plates of a parallel-plate capacitor, the electric field is zero inside the plates themselves, assuming ideal conditions (no edge effects).
2. **In a Superposition of Fields:**
- **Superposition Principle:** If multiple electric fields are present, the total electric field at any point is the vector sum of the individual fields. There can be points where the contributions from different fields cancel each other out, resulting in a net electric field of zero.
3. **In Conductors in Electrostatic Equilibrium:**
- **Inside a Conductor:** In electrostatic equilibrium, the electric field inside a perfect conductor is zero. This is because free charges within the conductor move in response to an electric field until it is neutralized.
4. **In Equipotential Surfaces:**
- **On Equipotential Surfaces:** If you are on a surface where the electric potential is constant (an equipotential surface), the electric field is perpendicular to this surface. In regions where this surface is flat and there are no other field contributions, the electric field can be zero in that flat region.
5. **In Some Specific Configurations:**
- **Conducting Spheres or Shells:** For spherical conductors, the electric field inside a hollow spherical conductor is zero if the conductor is in electrostatic equilibrium and no external fields are present.
These conditions are often used in solving problems involving electrostatics and fields.

Applied Physics

Signals and Systems

Digital Electronics
Basic Concepts
Basic Laws
Units

Ohmic Resistors
Capacitors and Inductors

RC Circuit
First-Order Circuits
Second-Order Circuits
Principles Of Circuit Analysis
Sinusoids and Phasors
AC Steady-State Analysis
Single Phase A.C. Circuits
Three-Phase Circuits
Resonance In Series And Parallel Circuits
Network Theorems
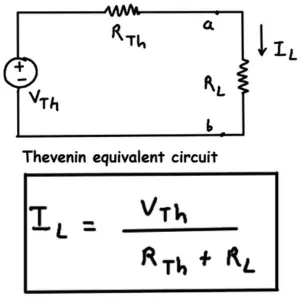
Thevenin's Theorem
Two-port Networks
Digital Electronics
Oscilloscope

Ohmmeter

Voltmeter
Ammeter

Induction Motor
Transformer
Operational Amplifiers
Components
Symbols
Formulas
EE Notes
EE Dictionary

MCQ Quiz

Interview Q&A
Power Electronics Book
Advanced Calculator

Basic Calculator
Simulator
Videos
Q&A

Capacitance Meter
Two Way Switch
Electrical Machines
Power Electronics

Electrical Drives & Their Control

Electrical Safety & Standards

Basics of Electronics Engineering
Electromagnetic Fields

Electrical Machines

More Items Coming Soon...