2 Answers
Here’s a step-by-step explanation of how to prove Gauss's Law:
### Gauss’s Law Statement
Gauss's Law states that the electric flux \(\Phi_E\) through a closed surface \(S\) is equal to the charge \(Q_{enc}\) enclosed by that surface divided by the permittivity of free space \(\epsilon_0\):
\[ \Phi_E = \frac{Q_{enc}}{\epsilon_0} \]
where the electric flux \(\Phi_E\) is given by:
\[ \Phi_E = \oint_S \mathbf{E} \cdot d\mathbf{A} \]
Here, \(\mathbf{E}\) is the electric field vector, and \(d\mathbf{A}\) is an infinitesimal vector area element on the surface \(S\).
### Proof Using Coulomb’s Law
1. **Start with Coulomb’s Law**:
For a point charge \(Q\) located at the origin, the electric field \(\mathbf{E}\) at a distance \(r\) from the charge is given by:
\[ \mathbf{E} = \frac{Q}{4 \pi \epsilon_0 r^2} \hat{r} \]
where \(\hat{r}\) is the unit vector in the radial direction.
2. **Choose a Gaussian Surface**:
To simplify the calculation, choose a spherical surface of radius \(r\) centered at the charge. This surface is known as a Gaussian surface. The symmetry of the problem (spherical symmetry) allows us to use Gauss’s Law effectively.
3. **Compute the Electric Flux**:
On a spherical Gaussian surface, the electric field \(\mathbf{E}\) is radially outward and has the same magnitude at every point on the surface. The infinitesimal area element \(d\mathbf{A}\) on the sphere is also radial. Therefore, \(\mathbf{E} \cdot d\mathbf{A} = E \cdot dA\), where \(E\) is the magnitude of the electric field.
The total electric flux \(\Phi_E\) through the Gaussian surface is:
\[ \Phi_E = \oint_S \mathbf{E} \cdot d\mathbf{A} \]
Since \(\mathbf{E}\) is constant in magnitude over the surface and radial:
\[ \Phi_E = E \oint_S dA \]
The surface area of a sphere of radius \(r\) is \(4 \pi r^2\):
\[ \Phi_E = E \cdot 4 \pi r^2 \]
Substituting \(E\) from Coulomb’s Law:
\[ \Phi_E = \frac{Q}{4 \pi \epsilon_0 r^2} \cdot 4 \pi r^2 \]
Simplify:
\[ \Phi_E = \frac{Q}{\epsilon_0} \]
4. **Generalize to Any Charge Distribution**:
For a general charge distribution, consider dividing the charge distribution into a large number of infinitesimal point charges \(dQ\). By applying the above spherical Gaussian surface approach to each infinitesimal charge and summing the contributions, you can show that the net flux through the surface is still given by the total enclosed charge divided by \(\epsilon_0\). This general principle extends to any symmetric charge distribution.
### Proof Using Divergence Theorem
1. **Apply the Divergence Theorem**:
The Divergence Theorem relates the flux of a vector field through a closed surface to the volume integral of the divergence of the field:
\[ \oint_S \mathbf{E} \cdot d\mathbf{A} = \int_V (\nabla \cdot \mathbf{E}) \, dV \]
where \(V\) is the volume enclosed by the surface \(S\).
2. **Use Gauss’s Law in Differential Form**:
Gauss's Law in differential form states:
\[ \nabla \cdot \mathbf{E} = \frac{\rho}{\epsilon_0} \]
where \(\rho\) is the charge density.
3. **Integrate Over the Volume**:
Substitute \(\nabla \cdot \mathbf{E}\) in the Divergence Theorem:
\[ \oint_S \mathbf{E} \cdot d\mathbf{A} = \int_V \frac{\rho}{\epsilon_0} \, dV \]
The integral of \(\rho\) over the volume \(V\) is the total charge \(Q_{enc}\) enclosed within the surface \(S\):
\[ \oint_S \mathbf{E} \cdot d\mathbf{A} = \frac{1}{\epsilon_0} \int_V \rho \, dV = \frac{Q_{enc}}{\epsilon_0} \]
This confirms Gauss’s Law.
### Summary
To prove Gauss’s Law, you can use either the direct approach with Coulomb’s Law and symmetry or the Divergence Theorem combined with the differential form of Gauss’s Law. Both methods show that the net electric flux through a closed surface is proportional to the total charge enclosed by that surface, validating the law as a fundamental principle of electromagnetism.
**Gauss's Law in Differential Form:**
\[ \nabla \cdot \mathbf{E} = \frac{\rho}{\epsilon_0} \]
where:
- \(\mathbf{E}\) is the electric field,
- \(\nabla \cdot \mathbf{E}\) is the divergence of the electric field,
- \(\rho\) is the charge density,
- \(\epsilon_0\) is the permittivity of free space.
**Gauss's Law in Integral Form:**
\[ \oint_{S} \mathbf{E} \cdot d\mathbf{A} = \frac{Q_{\text{enc}}}{\epsilon_0} \]
where:
- \( \oint_{S} \mathbf{E} \cdot d\mathbf{A} \) is the electric flux through a closed surface \( S \),
- \( Q_{\text{enc}} \) is the total charge enclosed within the surface \( S \).
**Steps to Prove Gauss's Law:**
1. **Start with Gauss’s Law in Differential Form:**
We have:
\[ \nabla \cdot \mathbf{E} = \frac{\rho}{\epsilon_0} \]
2. **Apply the Divergence Theorem:**
The Divergence Theorem relates the flux of a vector field through a closed surface to the volume integral of the divergence of the field. Mathematically, it states:
\[ \oint_{S} \mathbf{E} \cdot d\mathbf{A} = \int_{V} (\nabla \cdot \mathbf{E}) \, dV \]
where \( V \) is the volume enclosed by the surface \( S \).
3. **Substitute the Differential Form into the Divergence Theorem:**
Substitute \( \nabla \cdot \mathbf{E} = \frac{\rho}{\epsilon_0} \) into the Divergence Theorem:
\[ \oint_{S} \mathbf{E} \cdot d\mathbf{A} = \int_{V} \left( \frac{\rho}{\epsilon_0} \right) dV \]
4. **Simplify the Right Side of the Equation:**
Factor out \( \frac{1}{\epsilon_0} \) from the integral:
\[ \oint_{S} \mathbf{E} \cdot d\mathbf{A} = \frac{1}{\epsilon_0} \int_{V} \rho \, dV \]
5. **Recognize the Volume Integral of Charge Density:**
The volume integral \( \int_{V} \rho \, dV \) represents the total charge \( Q_{\text{enc}} \) enclosed within the volume \( V \):
\[ \int_{V} \rho \, dV = Q_{\text{enc}} \]
6. **Combine the Results:**
Substitute \( Q_{\text{enc}} \) back into the equation:
\[ \oint_{S} \mathbf{E} \cdot d\mathbf{A} = \frac{Q_{\text{enc}}}{\epsilon_0} \]
This is the integral form of Gauss’s Law, which we set out to prove.
**Summary:**
Gauss's Law in integral form states that the electric flux through a closed surface is proportional to the charge enclosed by that surface. By starting from the differential form of Gauss's Law and applying the Divergence Theorem, we can show that the integral form follows directly. This proves that Gauss's Law is consistent with the concept of electric flux and charge distribution in space.

Applied Physics

Signals and Systems

Digital Electronics
Basic Concepts
Basic Laws
Units

Ohmic Resistors
Capacitors and Inductors

RC Circuit
First-Order Circuits
Second-Order Circuits
Principles Of Circuit Analysis
Sinusoids and Phasors
AC Steady-State Analysis
Single Phase A.C. Circuits
Three-Phase Circuits
Resonance In Series And Parallel Circuits
Network Theorems
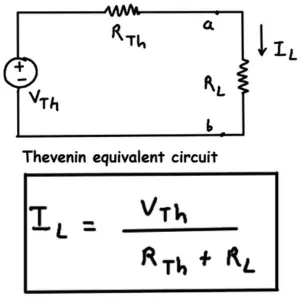
Thevenin's Theorem
Two-port Networks
Digital Electronics
Oscilloscope

Ohmmeter

Voltmeter
Ammeter

Induction Motor
Transformer
Operational Amplifiers
Components
Symbols
Formulas
EE Notes
EE Dictionary

MCQ Quiz

Interview Q&A
Power Electronics Book
Advanced Calculator

Basic Calculator
Simulator
Videos
Q&A

Capacitance Meter
Two Way Switch
Electrical Machines
Power Electronics

Electrical Drives & Their Control

Electrical Safety & Standards

Basics of Electronics Engineering
Electromagnetic Fields

Electrical Machines

More Items Coming Soon...