2 Answers
### **Statement of Gauss's Law:**
The law states:
\[
\oint \vec{E} \cdot d\vec{A} = \frac{Q_{\text{enc}}}{\varepsilon_0}
\]
Where:
- \(\vec{E}\) is the electric field.
- \(d\vec{A}\) is a differential area element on the closed surface, with an outward-facing normal vector.
- \(\oint\) denotes a surface integral over a closed surface.
- \(Q_{\text{enc}}\) is the total electric charge enclosed within the surface.
- \(\varepsilon_0\) is the permittivity of free space (\(8.854 \times 10^{-12} \, \text{F/m}\)).
### **Key Concepts:**
1. **Electric Flux**:
Electric flux is the amount of electric field passing through a given surface. Mathematically, it's the product of the electric field and the area perpendicular to the field:
\[
\Phi_E = \vec{E} \cdot A
\]
Gauss's law says that the total electric flux through a closed surface is proportional to the charge enclosed within that surface.
2. **Closed Surface**:
Gauss’s law is applied to a closed surface, often called a "Gaussian surface." This surface is an imaginary boundary that can take different shapes (like spheres, cylinders, or boxes), depending on the symmetry of the problem.
3. **Symmetry Consideration**:
Gauss’s law is particularly useful for calculating electric fields in situations with high symmetry, such as spherical, cylindrical, or planar symmetry. In these cases, the symmetry simplifies the mathematics and allows us to determine the electric field more easily.
4. **Permittivity of Free Space (\(\varepsilon_0\))**:
This is a constant that describes how electric fields interact in a vacuum. The value of \(\varepsilon_0\) is critical for calculations in Gauss's law.
### **Applications of Gauss's Law:**
1. **Electric Field of a Point Charge**:
Consider a point charge \(Q\). Using a spherical Gaussian surface centered around the charge, Gauss’s law helps in deriving Coulomb's law:
\[
E = \frac{Q}{4 \pi \varepsilon_0 r^2}
\]
2. **Electric Field of a Spherically Symmetric Charge Distribution**:
For a spherical shell or a uniformly charged sphere, Gauss’s law simplifies the electric field calculations inside and outside the charge distribution.
3. **Electric Field of an Infinite Line of Charge**:
For a long, charged wire, using a cylindrical Gaussian surface, Gauss’s law helps in deriving the electric field at a distance from the wire.
4. **Electric Field of a Uniformly Charged Plane**:
For a large, charged plane, Gauss’s law shows that the electric field is uniform near the surface.
### **Implications of Gauss's Law**:
- **Charge Inside a Conductor**:
In electrostatic equilibrium, the electric field inside a conductor is zero. Gauss's law helps explain why any excess charge on a conductor resides on its surface.
- **Faraday Cage**:
Gauss’s law explains why the electric field inside a hollow conductor (like a Faraday cage) is zero, protecting the interior from external electric fields.
### **Limitations**:
- Gauss's law is always true, but it is most useful in cases with symmetry. Without symmetry, calculating the electric field can be complex and less practical using Gauss's law alone.
In summary, **Gauss's law** provides a powerful tool to calculate electric fields in symmetrical charge distributions by linking the total electric flux to the enclosed charge.
### **Gauss's Law Statement:**
Gauss's Law states that the total electric flux through a closed surface is equal to the charge enclosed by that surface divided by the permittivity of free space (\(\epsilon_0\)).
Mathematically, Gauss's Law is expressed as:
\[ \Phi_E = \frac{Q_{enc}}{\epsilon_0} \]
where:
- \(\Phi_E\) is the electric flux through the closed surface.
- \(Q_{enc}\) is the total charge enclosed within the surface.
- \(\epsilon_0\) is the permittivity of free space (\(\epsilon_0 \approx 8.85 \times 10^{-12} \, \text{F/m}\)).
### **Electric Flux:**
Electric flux (\(\Phi_E\)) through a surface is given by:
\[ \Phi_E = \oint_S \mathbf{E} \cdot d\mathbf{A} \]
where:
- \(\mathbf{E}\) is the electric field vector.
- \(d\mathbf{A}\) is a differential area element of the surface \(S\).
- The integral is taken over the entire closed surface.
### **Applications of Gauss's Law:**
1. **Symmetric Charge Distributions:**
Gauss's Law is particularly useful for calculating electric fields in systems with high symmetry:
- **Spherical Symmetry:** For a point charge or a spherical charge distribution, use a spherical Gaussian surface.
- **Cylindrical Symmetry:** For an infinite line charge, use a cylindrical Gaussian surface.
- **Planar Symmetry:** For an infinite plane of charge, use a planar Gaussian surface.
2. **Conductors and Dielectrics:**
- **Inside a Conductor:** The electric field inside a perfect conductor is zero.
- **On the Surface of a Conductor:** The electric field just outside a charged conductor is perpendicular to the surface and proportional to the surface charge density.
3. **Gauss's Law in Differential Form:**
The differential form of Gauss's Law, which relates to Maxwell's equations, is:
\[ \nabla \cdot \mathbf{E} = \frac{\rho}{\epsilon_0} \]
where:
- \(\nabla \cdot \mathbf{E}\) is the divergence of the electric field.
- \(\rho\) is the charge density.
### **Important Points:**
- Gauss's Law is one of Maxwell's equations, which form the foundation of classical electromagnetism.
- It is applicable in both electrostatics and dynamic situations where charge distributions are changing.
- The law is a direct consequence of the conservation of electric charge and is derived from Coulomb's Law.
### **Example Problems:**
1. **Point Charge:** For a point charge \(Q\) at the center of a spherical Gaussian surface of radius \(r\), the electric flux is:
\[ \Phi_E = \frac{Q}{\epsilon_0} \]
2. **Infinite Line Charge:** For an infinite line charge with linear charge density \(\lambda\), using a cylindrical Gaussian surface of radius \(r\) and length \(L\), the electric field \(E\) is:
\[ E = \frac{\lambda}{2 \pi \epsilon_0 r} \]
3. **Infinite Plane Charge:** For an infinite plane with surface charge density \(\sigma\), the electric field \(E\) is:
\[ E = \frac{\sigma}{2 \epsilon_0} \]
Gauss's Law provides a powerful method for solving electrostatic problems, especially when dealing with symmetrical charge distributions. It simplifies the calculation of electric fields and helps to understand the behavior of electric fields in different scenarios.

Applied Physics

Signals and Systems

Digital Electronics
Basic Concepts
Basic Laws
Units

Ohmic Resistors
Capacitors and Inductors

RC Circuit
First-Order Circuits
Second-Order Circuits
Principles Of Circuit Analysis
Sinusoids and Phasors
AC Steady-State Analysis
Single Phase A.C. Circuits
Three-Phase Circuits
Resonance In Series And Parallel Circuits
Network Theorems
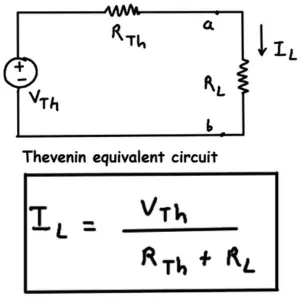
Thevenin's Theorem
Two-port Networks
Digital Electronics
Oscilloscope

Ohmmeter

Voltmeter
Ammeter

Induction Motor
Transformer
Operational Amplifiers
Components
Symbols
Formulas
EE Notes
EE Dictionary

MCQ Quiz

Interview Q&A
Power Electronics Book
Advanced Calculator

Basic Calculator
Simulator
Videos
Q&A

Capacitance Meter
Two Way Switch
Electrical Machines
Power Electronics

Electrical Drives & Their Control

Electrical Safety & Standards

Basics of Electronics Engineering
Electromagnetic Fields

Electrical Machines

More Items Coming Soon...