2 Answers
### Mathematical Formulation
In one dimension, the Laplace equation is written as:
\[ \frac{d^2 u(x)}{dx^2} = 0 \]
where \( u(x) \) is a function of the spatial variable \( x \). This equation states that the second derivative of \( u \) with respect to \( x \) is zero.
### Interpretation
1. **Physical Meaning**: In physical terms, this equation often arises in steady-state problems where the distribution of a quantity (like temperature, electric potential, etc.) does not change with time. The condition that the second derivative is zero implies that the quantity \( u(x) \) changes linearly with \( x \). So, the general solution to this equation is a linear function:
\[ u(x) = Ax + B \]
where \( A \) and \( B \) are constants determined by boundary conditions.
2. **Boundary Conditions**: To fully solve a problem involving the one-dimensional Laplace equation, you need boundary conditions. These might specify the value of \( u(x) \) at certain points, or the rate of change of \( u(x) \) at these points. For example:
- **Dirichlet Boundary Conditions**: Specify the value of \( u \) at the boundaries.
- **Neumann Boundary Conditions**: Specify the value of the derivative of \( u \) (i.e., the gradient) at the boundaries.
### Example
Consider a rod of length \( L \) with ends at \( x = 0 \) and \( x = L \). Suppose the temperature at \( x = 0 \) is \( T_0 \) and at \( x = L \) is \( T_L \). Using the one-dimensional Laplace equation, the temperature distribution \( T(x) \) in the rod would be a linear function:
\[ T(x) = \frac{T_L - T_0}{L} x + T_0 \]
Here, the slope of the temperature distribution is determined by the difference in temperatures and the length of the rod.
### Summary
The one-dimensional Laplace equation \(\frac{d^2 u}{dx^2} = 0\) describes scenarios where the quantity of interest (such as temperature, potential, or concentration) varies linearly along a single spatial dimension. It’s a simplified but fundamental case of the more general Laplace equation used in higher dimensions.
### Mathematical Form
In one dimension, the Laplace equation is written as:
\[
\frac{d^2 u(x)}{dx^2} = 0
\]
Here:
- \( u(x) \) is a function of the spatial variable \( x \), which we are trying to solve for.
- \( \frac{d^2 u}{dx^2} \) represents the second derivative of \( u(x) \) with respect to \( x \).
### Meaning of the Equation
This equation tells us that the second derivative of the function \( u(x) \) is zero at every point in the domain. This implies that the function \( u(x) \) does not change its curvature as you move along the x-axis — meaning the function is linear.
### General Solution
The general solution to the one-dimensional Laplace equation is obtained by integrating twice:
\[
u(x) = Ax + B
\]
Where:
- \( A \) and \( B \) are constants determined by the boundary conditions of the problem.
### Physical Interpretation
The one-dimensional Laplace equation is used to model scenarios where there is no change in the distribution of a quantity over time. Examples include:
- **Heat conduction in a steady state**: where the temperature distribution doesn't change with time.
- **Electrostatic potential in free space**: where there are no sources of electric charge.
In both cases, the solution describes a system that has reached equilibrium, and the distribution of the temperature or electric potential is linear along the one-dimensional domain.
### Example: Solving with Boundary Conditions
Suppose you have a rod of length \( L \) with boundary conditions:
- \( u(0) = u_0 \)
- \( u(L) = u_L \)
You solve the Laplace equation \( \frac{d^2 u}{dx^2} = 0 \) by integrating it, giving the general solution \( u(x) = Ax + B \). To find the constants \( A \) and \( B \), you use the boundary conditions:
1. \( u(0) = B = u_0 \)
2. \( u(L) = AL + u_0 = u_L \)
From the second equation, you solve for \( A \):
\[
A = \frac{u_L - u_0}{L}
\]
So the solution is:
\[
u(x) = \frac{u_L - u_0}{L}x + u_0
\]
This represents a linear distribution of \( u(x) \) (e.g., temperature or potential) along the rod.
### Conclusion
The one-dimensional Laplace equation is a simple yet powerful equation that describes how certain physical quantities distribute themselves in space when in a steady state, with no time dependence or sources in the domain. The solution is always a straight line determined by the boundary conditions of the problem.

Applied Physics

Signals and Systems

Digital Electronics
Basic Concepts
Basic Laws
Units

Ohmic Resistors
Capacitors and Inductors

RC Circuit
First-Order Circuits
Second-Order Circuits
Principles Of Circuit Analysis
Sinusoids and Phasors
AC Steady-State Analysis
Single Phase A.C. Circuits
Three-Phase Circuits
Resonance In Series And Parallel Circuits
Network Theorems
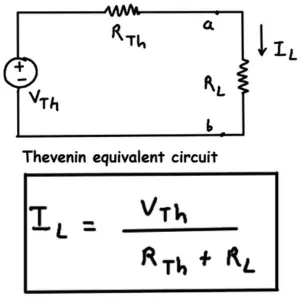
Thevenin's Theorem
Two-port Networks
Digital Electronics
Oscilloscope

Ohmmeter

Voltmeter
Ammeter

Induction Motor
Transformer
Operational Amplifiers
Components
Symbols
Formulas
EE Notes
EE Dictionary

MCQ Quiz

Interview Q&A
Power Electronics Book
Advanced Calculator

Basic Calculator
Simulator
Videos
Q&A

Capacitance Meter
Two Way Switch
Electrical Machines
Power Electronics

Electrical Drives & Their Control

Electrical Safety & Standards

Basics of Electronics Engineering
Electromagnetic Fields

Electrical Machines

More Items Coming Soon...