2 Answers
### Definition
The Laplace transform \( \mathcal{L} \) of a function \( f(t) \) is defined by the following integral:
\[ \mathcal{L}\{f(t)\} = F(s) = \int_0^\infty f(t) e^{-st} \, dt \]
where:
- \( t \) is the time variable,
- \( s \) is a complex number frequency parameter (\( s = \sigma + j\omega \)),
- \( f(t) \) is the time-domain function,
- \( F(s) \) is the transformed function in the Laplace domain.
### Steps to Compute the Laplace Transform
1. **Identify the Function \( f(t) \)**:
- Determine the function you want to transform. This function should be defined for \( t \geq 0 \).
2. **Set Up the Integral**:
- Substitute \( f(t) \) into the Laplace transform formula:
\[
\mathcal{L}\{f(t)\} = \int_0^\infty f(t) e^{-st} \, dt
\]
3. **Evaluate the Integral**:
- Compute the integral to find \( F(s) \). This often involves integration techniques and might require some manipulation depending on the complexity of \( f(t) \).
4. **Simplify the Result**:
- Simplify \( F(s) \) to get it in a standard or more usable form.
### Examples
1. **Example 1: Exponential Function**
For \( f(t) = e^{at} \):
\[
\mathcal{L}\{e^{at}\} = \int_0^\infty e^{at} e^{-st} \, dt = \int_0^\infty e^{(a-s)t} \, dt
\]
To solve this:
\[
\int_0^\infty e^{(a-s)t} \, dt = \left[ \frac{e^{(a-s)t}}{a-s} \right]_0^\infty
\]
Assuming \( \text{Re}(s) > \text{Re}(a) \), this evaluates to:
\[
\mathcal{L}\{e^{at}\} = \frac{1}{s-a}
\]
2. **Example 2: Sine Function**
For \( f(t) = \sin(bt) \):
\[
\mathcal{L}\{\sin(bt)\} = \int_0^\infty \sin(bt) e^{-st} \, dt
\]
To solve this:
\[
\int_0^\infty \sin(bt) e^{-st} \, dt = \frac{b}{s^2 + b^2}
\]
Thus:
\[
\mathcal{L}\{\sin(bt)\} = \frac{b}{s^2 + b^2}
\]
### Common Laplace Transforms
- **Constant Function \( f(t) = 1 \)**:
\[
\mathcal{L}\{1\} = \frac{1}{s}
\]
- **Unit Step Function \( f(t) = u(t) \)**:
\[
\mathcal{L}\{u(t)\} = \frac{1}{s}
\]
- **Delta Function \( f(t) = \delta(t) \)**:
\[
\mathcal{L}\{\delta(t)\} = 1
\]
- **Derivative of a Function**:
If \( \mathcal{L}\{f(t)\} = F(s) \), then:
\[
\mathcal{L}\{f'(t)\} = sF(s) - f(0)
\]
Understanding and using the Laplace transform can greatly simplify the process of solving differential equations and analyzing system behaviors.
Here's a detailed breakdown of how to write and compute a Laplace transform:
### 1. **Understanding the Definition**
The Laplace transform \( \mathcal{L}\{f(t)\} \) of a function \( f(t) \) is defined by the integral:
\[ \mathcal{L}\{f(t)\} = F(s) = \int_{0}^{\infty} e^{-st} f(t) \, dt \]
where:
- \( t \) is the time variable (usually \( t \geq 0 \)),
- \( s \) is a complex variable (usually \( s = \sigma + j\omega \), where \( \sigma \) and \( \omega \) are real numbers, and \( j \) is the imaginary unit),
- \( F(s) \) is the resulting function in the \( s \)-domain.
### 2. **Applying the Definition**
To apply the definition, follow these steps:
- **Identify the function \( f(t) \)**: This is the time-domain function you want to transform.
- **Set up the integral**: Substitute \( f(t) \) into the integral formula \( \int_{0}^{\infty} e^{-st} f(t) \, dt \).
- **Evaluate the integral**: Perform the integration with respect to \( t \).
### 3. **Example**
Let’s compute the Laplace transform of a simple function, \( f(t) = e^{at} \), where \( a \) is a constant.
Using the definition:
\[ \mathcal{L}\{e^{at}\} = \int_{0}^{\infty} e^{-st} e^{at} \, dt \]
Combine the exponents:
\[ = \int_{0}^{\infty} e^{(a - s)t} \, dt \]
This is an exponential integral. For convergence, \( \text{Re}(s) > a \). Integrate:
\[ \int_{0}^{\infty} e^{(a - s)t} \, dt = \left[ \frac{e^{(a - s)t}}{a - s} \right]_{0}^{\infty} \]
Evaluating the integral:
- At \( t = \infty \), \( e^{(a - s)t} \) approaches 0 if \( \text{Re}(s) > a \).
- At \( t = 0 \), \( e^{(a - s)t} = 1 \).
Thus:
\[ \mathcal{L}\{e^{at}\} = \frac{1}{s - a} \]
### 4. **Common Laplace Transforms**
Here are some standard transforms you might find useful:
- **Constant function** \( f(t) = 1 \): \( \mathcal{L}\{1\} = \frac{1}{s} \)
- **Power function** \( f(t) = t^n \): \( \mathcal{L}\{t^n\} = \frac{n!}{s^{n+1}} \)
- **Sine function** \( f(t) = \sin(\omega t) \): \( \mathcal{L}\{\sin(\omega t)\} = \frac{\omega}{s^2 + \omega^2} \)
- **Cosine function** \( f(t) = \cos(\omega t) \): \( \mathcal{L}\{\cos(\omega t)\} = \frac{s}{s^2 + \omega^2} \)
### 5. **Inverse Laplace Transform**
To find the original function \( f(t) \) from \( F(s) \), you use the inverse Laplace transform, denoted \( \mathcal{L}^{-1}\{F(s)\} \). The inverse transform is not always straightforward but follows similar principles as the Laplace transform.
### Summary
To write and compute a Laplace transform, you:
1. Identify the time-domain function \( f(t) \).
2. Set up and evaluate the integral \( \int_{0}^{\infty} e^{-st} f(t) \, dt \).
3. Simplify the result to find \( F(s) \).
Understanding and applying these steps allows you to transform functions between the time domain and the complex \( s \)-domain, which is particularly helpful for solving linear differential equations and analyzing systems.

Applied Physics

Signals and Systems

Digital Electronics
Basic Concepts
Basic Laws
Units

Ohmic Resistors
Capacitors and Inductors

RC Circuit
First-Order Circuits
Second-Order Circuits
Principles Of Circuit Analysis
Sinusoids and Phasors
AC Steady-State Analysis
Single Phase A.C. Circuits
Three-Phase Circuits
Resonance In Series And Parallel Circuits
Network Theorems
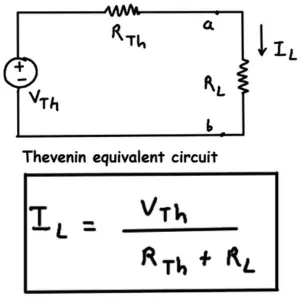
Thevenin's Theorem
Two-port Networks
Digital Electronics
Oscilloscope

Ohmmeter

Voltmeter
Ammeter

Induction Motor
Transformer
Operational Amplifiers
Components
Symbols
Formulas
EE Notes
EE Dictionary

MCQ Quiz

Interview Q&A
Power Electronics Book
Advanced Calculator

Basic Calculator
Simulator
Videos
Q&A

Capacitance Meter
Two Way Switch
Electrical Machines
Power Electronics

Electrical Drives & Their Control

Electrical Safety & Standards

Basics of Electronics Engineering
Electromagnetic Fields

Electrical Machines

More Items Coming Soon...