2 Answers
\[
\frac{\Delta G}{T} = -\frac{\Delta H}{T^2} + \text{constant}
\]
Where:
- \( \Delta G \) is the change in Gibbs free energy of the system.
- \( \Delta H \) is the change in enthalpy of the system.
- \( T \) is the absolute temperature (measured in Kelvin).
- The constant term depends on the specifics of the system and conditions.
### Explanation of Terms
1. **Gibbs Free Energy (\( G \))**:
- Gibbs free energy is a thermodynamic potential that measures the maximum reversible work obtainable from a system at constant temperature and pressure. It is defined as:
\[
G = H - TS
\]
where \( H \) is enthalpy and \( S \) is entropy.
2. **Enthalpy (\( H \))**:
- Enthalpy is a measure of the total energy of a thermodynamic system, including internal energy and the energy required to make room for it by displacing its environment. It is defined as:
\[
H = U + PV
\]
where \( U \) is internal energy, \( P \) is pressure, and \( V \) is volume.
3. **Entropy (\( S \))**:
- Entropy is a measure of the disorder or randomness in a system, and it also reflects the amount of energy in a physical system that is not available to do work.
### Importance of the Gibbs-Helmholtz Equation
- **Thermodynamic Equilibrium**: The Gibbs-Helmholtz equation helps in understanding the conditions under which a reaction will occur. A negative value of \( \Delta G \) indicates that a process is spontaneous.
- **Phase Changes**: The equation is particularly useful for analyzing phase changes and chemical reactions at constant temperature and pressure. It provides insights into how changes in temperature affect the spontaneity of reactions.
- **Calculating Thermodynamic Properties**: It allows for the calculation of changes in Gibbs free energy and helps predict how a system will respond to changes in temperature.
### Applications
1. **Chemical Reactions**: In chemistry, the Gibbs-Helmholtz equation is used to determine whether a reaction will proceed spontaneously under certain conditions.
2. **Phase Equilibria**: It can be applied in studying phase transitions, like melting or boiling, helping to determine the temperature and pressure at which phases coexist.
3. **Biochemical Processes**: In biological systems, it assists in understanding metabolic reactions and energy transfers.
### Conclusion
In summary, the Gibbs-Helmholtz equation is a crucial thermodynamic relationship that links free energy, enthalpy, and temperature, providing insights into the spontaneity and feasibility of chemical and physical processes. Understanding this equation is essential for both theoretical and practical applications in chemistry, physics, and engineering.
The equation can be expressed as:
\[ \left( \frac{\partial G}{\partial T} \right)_P = -S \]
where:
- \( G \) is the Gibbs free energy,
- \( T \) is the temperature,
- \( S \) is the entropy,
- \( \left( \frac{\partial G}{\partial T} \right)_P \) represents the partial derivative of Gibbs free energy with respect to temperature at constant pressure.
### Breakdown of the Equation
1. **Gibbs Free Energy (G)**:
- Gibbs free energy combines the system's enthalpy and entropy to predict the spontaneity of processes. It is given by:
\[ G = H - TS \]
where \( H \) is the enthalpy, \( T \) is the temperature, and \( S \) is the entropy.
2. **Partial Derivative of G with Respect to T**:
- The Gibbs-Helmholtz equation tells us that the rate of change of Gibbs free energy with temperature, under constant pressure, is equal to the negative entropy of the system. This relationship helps us understand how the Gibbs free energy—and hence the spontaneity of a process—varies with temperature.
3. **Entropy (S)**:
- Entropy is a measure of the disorder or randomness in a system. In the context of the Gibbs-Helmholtz equation, it provides insight into how the disorder changes with temperature.
### Implications
- **Temperature Dependence**:
The equation shows that if entropy \( S \) is positive, the Gibbs free energy \( G \) decreases as temperature increases, and vice versa. This has important implications for chemical reactions and phase transitions. For instance, if a reaction is endothermic (absorbs heat), the entropy change can drive the reaction to become spontaneous at higher temperatures.
- **Practical Applications**:
The Gibbs-Helmholtz equation is useful in various practical applications, such as predicting the temperature at which a reaction becomes spontaneous or understanding phase diagrams. It helps chemists and engineers optimize conditions for reactions and processes.
In summary, the Gibbs-Helmholtz equation is a fundamental relationship in thermodynamics that links the change in Gibbs free energy with temperature and entropy, providing crucial insights into the behavior of systems under varying thermal conditions.

Applied Physics

Signals and Systems

Digital Electronics
Basic Concepts
Basic Laws
Units

Ohmic Resistors
Capacitors and Inductors

RC Circuit
First-Order Circuits
Second-Order Circuits
Principles Of Circuit Analysis
Sinusoids and Phasors
AC Steady-State Analysis
Single Phase A.C. Circuits
Three-Phase Circuits
Resonance In Series And Parallel Circuits
Network Theorems
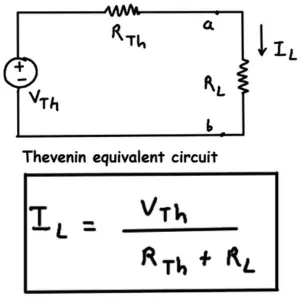
Thevenin's Theorem
Two-port Networks
Digital Electronics
Oscilloscope

Ohmmeter

Voltmeter
Ammeter

Induction Motor
Transformer
Operational Amplifiers
Components
Symbols
Formulas
EE Notes
EE Dictionary

MCQ Quiz

Interview Q&A
Power Electronics Book
Advanced Calculator

Basic Calculator
Simulator
Videos
Q&A

Capacitance Meter
Two Way Switch
Electrical Machines
Power Electronics

Electrical Drives & Their Control

Electrical Safety & Standards

Basics of Electronics Engineering
Electromagnetic Fields

Electrical Machines

More Items Coming Soon...