2 Answers
### Fundamental Principles
1. **Refraction**:
- Refraction is the bending of light as it passes from one medium to another with a different density (like air to water).
- This bending occurs because light travels at different speeds in different materials.
2. **Speed of Light**:
- The speed of light is fastest in a vacuum (approximately \(299,792,458\) meters per second) and slows down when it travels through materials like air, water, or glass.
- The ratio of the speed of light in a vacuum (\(c\)) to its speed in a given medium (\(v\)) is known as the refractive index (\(n\)):
\[
n = \frac{c}{v}
\]
- Different materials have different refractive indices, which affects how much light bends when entering or exiting the material.
3. **Angles of Incidence and Refraction**:
- When a light ray hits the boundary between two media, it creates two angles: the angle of incidence (\(θ_1\)), which is the angle between the incoming ray and the normal (an imaginary line perpendicular to the surface), and the angle of refraction (\(θ_2\)), which is the angle between the refracted ray and the normal.
### Snell’s Law Equation
Snell’s Law mathematically relates the angles of incidence and refraction to the refractive indices of the two media:
\[
n_1 \sin(θ_1) = n_2 \sin(θ_2)
\]
Where:
- \(n_1\) = refractive index of the first medium
- \(n_2\) = refractive index of the second medium
- \(θ_1\) = angle of incidence
- \(θ_2\) = angle of refraction
### Interpretation of Snell’s Law
1. **Critical Angle and Total Internal Reflection**:
- If light travels from a denser medium (higher refractive index) to a less dense medium (lower refractive index), there exists a critical angle beyond which all light is reflected back into the denser medium. This phenomenon is known as total internal reflection.
- The critical angle (\(θ_c\)) can be calculated using:
\[
\sin(θ_c) = \frac{n_2}{n_1}
\]
- For example, if light travels from water (\(n \approx 1.33\)) to air (\(n \approx 1.00\)), the critical angle can be calculated, and any incident angle greater than this will result in total internal reflection.
2. **Applications of Snell’s Law**:
- **Optics**: Snell’s Law is fundamental in designing lenses, prisms, and optical fibers. It helps in understanding how light behaves in different materials and is crucial for applications like glasses, cameras, and microscopes.
- **Seismology**: The law is used to study seismic waves as they travel through different layers of the Earth.
- **Fiber Optics**: It enables the design of optical fibers, allowing data transmission with minimal loss.
### Example Calculation
To illustrate how Snell's Law works, consider a light ray traveling from air into water:
- Let’s assume:
- \(n_1\) (air) = 1.00
- \(n_2\) (water) = 1.33
- The angle of incidence (\(θ_1\)) = 30 degrees
Using Snell's Law:
\[
1.00 \sin(30^\circ) = 1.33 \sin(θ_2)
\]
Calculating \( \sin(30^\circ) = 0.5 \):
\[
1.00 \cdot 0.5 = 1.33 \sin(θ_2)
\]
\[
0.5 = 1.33 \sin(θ_2)
\]
\[
\sin(θ_2) = \frac{0.5}{1.33} \approx 0.376
\]
Calculating the angle of refraction (\(θ_2\)):
\[
θ_2 \approx \arcsin(0.376) \approx 22.1^\circ
\]
### Conclusion
Snell’s Law is a fundamental principle in optics that describes the behavior of light as it transitions between different media. Understanding this law is crucial for applications across various scientific and engineering fields, providing insights into the nature of light and its interactions with materials.
### Basis of Snell's Law
Snell's Law is based on the principle of **conservation of energy** and **Huygens' principle**, which states that every point on a wavefront can be considered a source of secondary wavelets. Here's a detailed look at the concepts involved:
1. **Refraction**: When light travels from one medium to another (e.g., from air into water), its speed changes due to the differing optical densities of the media. This change in speed causes the light to bend. Snell's Law quantifies this bending.
2. **Refractive Index**: Each medium has a refractive index, denoted by \( n \), which measures how much the speed of light is reduced in that medium compared to its speed in a vacuum. The refractive index \( n \) is given by:
\[
n = \frac{c}{v}
\]
where \( c \) is the speed of light in a vacuum, and \( v \) is the speed of light in the medium.
3. **Snell's Law Formula**: The relationship between the angles of incidence and refraction and the refractive indices of the two media is expressed by:
\[
\frac{\sin \theta_1}{\sin \theta_2} = \frac{n_2}{n_1}
\]
where:
- \( \theta_1 \) is the angle of incidence (the angle between the incident ray and the normal to the surface),
- \( \theta_2 \) is the angle of refraction (the angle between the refracted ray and the normal),
- \( n_1 \) is the refractive index of the first medium,
- \( n_2 \) is the refractive index of the second medium.
### Derivation
Snell's Law can be derived using Huygens' principle, which states that every point on a wavefront is a source of secondary wavelets. When these wavelets enter a new medium at an angle, they travel at different speeds, causing the wavefront to bend. The geometry of the wavefronts' movement in the two media leads to Snell's Law.
### Practical Implications
Snell's Law is crucial in various applications, including:
- **Optical Design**: It helps in designing lenses and optical instruments by predicting how light will bend through different materials.
- **Fiber Optics**: It is used to understand how light signals are transmitted through optical fibers.
- **Atmospheric Science**: It explains phenomena like the bending of light around the Earth, which affects how we see distant objects.
In summary, Snell's Law is based on the fundamental principles of how light interacts with different media and how energy is conserved during this interaction.

Applied Physics

Signals and Systems

Digital Electronics
Basic Concepts
Basic Laws
Units

Ohmic Resistors
Capacitors and Inductors

RC Circuit
First-Order Circuits
Second-Order Circuits
Principles Of Circuit Analysis
Sinusoids and Phasors
AC Steady-State Analysis
Single Phase A.C. Circuits
Three-Phase Circuits
Resonance In Series And Parallel Circuits
Network Theorems
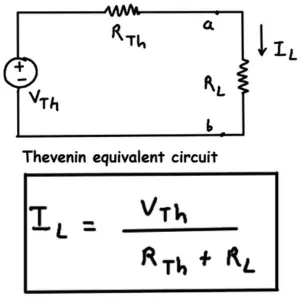
Thevenin's Theorem
Two-port Networks
Digital Electronics
Oscilloscope

Ohmmeter

Voltmeter
Ammeter

Induction Motor
Transformer
Operational Amplifiers
Components
Symbols
Formulas
EE Notes
EE Dictionary

MCQ Quiz

Interview Q&A
Power Electronics Book
Advanced Calculator

Basic Calculator
Simulator
Videos
Q&A

Capacitance Meter
Two Way Switch
Electrical Machines
Power Electronics

Electrical Drives & Their Control

Electrical Safety & Standards

Basics of Electronics Engineering
Electromagnetic Fields

Electrical Machines

More Items Coming Soon...