2 Answers
### 1. **Einstein's Field Equations**
Einstein's field equations (EFE) are the cornerstone of Einstein's theory of general relativity. They describe how matter and energy influence the curvature of spacetime, which we perceive as gravity. The equations are:
\[ G_{\mu\nu} = \frac{8\pi G}{c^4} T_{\mu\nu} \]
Here:
- \( G_{\mu\nu} \) is the Einstein tensor, which represents the curvature of spacetime.
- \( T_{\mu\nu} \) is the stress-energy tensor, which represents the distribution of matter and energy.
- \( G \) is the gravitational constant.
- \( c \) is the speed of light.
### 2. **Maxwell's Equations**
Maxwell's equations describe how electric and magnetic fields interact and propagate through space. They consist of four fundamental equations:
1. **Gauss's Law for Electricity:**
\[ \nabla \cdot \mathbf{E} = \frac{\rho}{\epsilon_0} \]
2. **Gauss's Law for Magnetism:**
\[ \nabla \cdot \mathbf{B} = 0 \]
3. **Faraday's Law of Induction:**
\[ \nabla \times \mathbf{E} = -\frac{\partial \mathbf{B}}{\partial t} \]
4. **Ampère's Law with Maxwell's Addition:**
\[ \nabla \times \mathbf{B} = \mu_0 \mathbf{J} + \mu_0 \epsilon_0 \frac{\partial \mathbf{E}}{\partial t} \]
Here:
- \( \mathbf{E} \) is the electric field.
- \( \mathbf{B} \) is the magnetic field.
- \( \rho \) is the electric charge density.
- \( \mathbf{J} \) is the current density.
- \( \epsilon_0 \) is the electric constant (permittivity of free space).
- \( \mu_0 \) is the magnetic constant (permeability of free space).
### 3. **Combining Einstein's Field Equations with Maxwell's Equations**
In the presence of electromagnetic fields, the stress-energy tensor \( T_{\mu\nu} \) in Einstein's field equations includes contributions from the electromagnetic field. The electromagnetic stress-energy tensor is given by:
\[ T_{\mu\nu}^{\text{em}} = \frac{1}{\mu_0} \left( F_{\mu\alpha} F_{\nu}^{\ \alpha} - \frac{1}{4} g_{\mu\nu} F_{\alpha\beta} F^{\alpha\beta} \right) \]
where \( F_{\mu\nu} \) is the electromagnetic field tensor and \( g_{\mu\nu} \) is the metric tensor describing the geometry of spacetime.
### 4. **The Einstein-Maxwell Equations**
In essence, when you combine these elements, you get the Einstein-Maxwell equations, which describe how electromagnetic fields influence the curvature of spacetime. These equations are particularly important in astrophysics and cosmology, where strong gravitational and electromagnetic fields interact, such as in the vicinity of black holes or neutron stars.
In summary:
- **Einstein's Field Equations** describe how matter and energy curve spacetime.
- **Maxwell's Equations** describe how electric and magnetic fields propagate and interact.
- **Einstein-Maxwell Equations** combine these frameworks to describe interactions between electromagnetic fields and curved spacetime.
These equations provide a unified way to understand complex interactions in the universe, where both strong gravitational fields and electromagnetic forces play significant roles.

Applied Physics

Signals and Systems

Digital Electronics
Basic Concepts
Basic Laws
Units

Ohmic Resistors
Capacitors and Inductors

RC Circuit
First-Order Circuits
Second-Order Circuits
Principles Of Circuit Analysis
Sinusoids and Phasors
AC Steady-State Analysis
Single Phase A.C. Circuits
Three-Phase Circuits
Resonance In Series And Parallel Circuits
Network Theorems
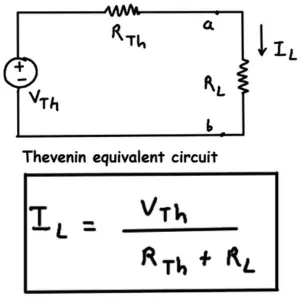
Thevenin's Theorem
Two-port Networks
Digital Electronics
Oscilloscope

Ohmmeter

Voltmeter
Ammeter

Induction Motor
Transformer
Operational Amplifiers
Components
Symbols
Formulas
EE Notes
EE Dictionary

MCQ Quiz

Interview Q&A
Power Electronics Book
Advanced Calculator

Basic Calculator
Simulator
Videos
Q&A

Capacitance Meter
Two Way Switch
Electrical Machines
Power Electronics

Electrical Drives & Their Control

Electrical Safety & Standards

Basics of Electronics Engineering
Electromagnetic Fields

Electrical Machines

More Items Coming Soon...