2 Answers
### Key Concept: LC Circuit
An LC circuit is a resonant circuit where the inductor and capacitor exchange energy, leading to oscillations. The inductor stores energy in its magnetic field, while the capacitor stores energy in its electric field. These oscillations occur at a natural resonant frequency.
#### 1. **Natural Frequency of an LC Circuit**:
The oscillation in an LC circuit happens at the resonant frequency, \( \omega_0 \), given by:
\[
\omega_0 = \frac{1}{\sqrt{LC}}
\]
where:
- \( \omega_0 \) is the angular frequency in radians per second,
- \( L \) is the inductance of the inductor (in henries, H),
- \( C \) is the capacitance of the capacitor (in farads, F).
If you want the **frequency** in hertz (cycles per second), it is:
\[
f_0 = \frac{1}{2\pi \sqrt{LC}}
\]
#### 2. **Oscillation Period**:
The period \( T \), or the time for one complete cycle of oscillation, is the inverse of the frequency:
\[
T = \frac{1}{f_0} = 2\pi \sqrt{LC}
\]
This is essentially the characteristic timing for an LC circuit, as it tells you how long one cycle of oscillation takes.
### Time Constant in the Context of LC Circuits
Unlike RC or RL circuits, which decay over time due to resistive losses and can be characterized by a **time constant** (where the system's response decays exponentially), **ideal LC circuits** oscillate forever without damping in a sinusoidal fashion. In such an ideal scenario, there is **no true time constant** in the traditional sense because the circuit is **undamped** and continues oscillating indefinitely.
However, if we consider **non-ideal LC circuits**, where resistive losses are present (due to parasitic resistance \( R \) in the inductor or wires), the oscillations will gradually decay. The time constant for this decay is related to the **damping factor** and is part of the concept of a **damped oscillation**.
For a real-world **RLC circuit** (where resistance is added), the system's behavior can be underdamped, critically damped, or overdamped. In this case, the time constant \( \tau \) is influenced by the resistance \( R \), inductance \( L \), and capacitance \( C \).
---
### Summary:
- In an **ideal LC circuit**, there is no true time constant since it oscillates indefinitely at a natural frequency.
- The **period** \( T \) of oscillation is \( T = 2\pi \sqrt{LC} \), which gives the characteristic timing.
- For a **non-ideal LC circuit** (or RLC circuit with resistance), oscillations are damped, and a time constant related to the damping can be defined, but this involves the resistance \( R \) in the circuit.
In an LC circuit, the behavior is characterized by oscillatory responses rather than exponential decay or growth. The key parameter for an LC circuit is the **resonant frequency**, which is given by:
\[ \omega_0 = \frac{1}{\sqrt{LC}} \]
where:
- \( \omega_0 \) is the angular resonant frequency (in radians per second),
- \( L \) is the inductance (in henries, H),
- \( C \) is the capacitance (in farads, F).
The resonant frequency \( f_0 \) in hertz (Hz) can be found from:
\[ f_0 = \frac{1}{2\pi\sqrt{LC}} \]
In terms of time, the period \( T \) of the oscillation, which is the time it takes for the circuit to complete one cycle, is:
\[ T = \frac{1}{f_0} = 2\pi\sqrt{LC} \]
While this period describes the oscillation cycle, it is not a time constant in the same sense as for RC or RL circuits, where the time constant \( \tau \) is used to describe how quickly the circuit responds to changes. For an LC circuit, the behavior is defined by its resonant frequency and the oscillation period.

Applied Physics

Signals and Systems

Digital Electronics
Basic Concepts
Basic Laws
Units

Ohmic Resistors
Capacitors and Inductors

RC Circuit
First-Order Circuits
Second-Order Circuits
Principles Of Circuit Analysis
Sinusoids and Phasors
AC Steady-State Analysis
Single Phase A.C. Circuits
Three-Phase Circuits
Resonance In Series And Parallel Circuits
Network Theorems
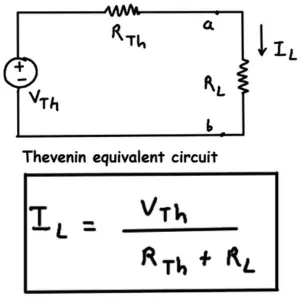
Thevenin's Theorem
Two-port Networks
Digital Electronics
Oscilloscope

Ohmmeter

Voltmeter
Ammeter

Induction Motor
Transformer
Operational Amplifiers
Components
Symbols
Formulas
EE Notes
EE Dictionary

MCQ Quiz

Interview Q&A
Power Electronics Book
Advanced Calculator

Basic Calculator
Simulator
Videos
Q&A

Capacitance Meter
Two Way Switch
Electrical Machines
Power Electronics

Electrical Drives & Their Control

Electrical Safety & Standards

Basics of Electronics Engineering
Electromagnetic Fields

Electrical Machines

More Items Coming Soon...