2 Answers
### Key Components of an LC Oscillator:
1. **Inductor (L)**: This component stores energy in the form of a magnetic field when current flows through it.
2. **Capacitor (C)**: This stores energy in the form of an electric field when it is charged by an applied voltage.
When these components are connected together, they form an LC circuit, which has the potential to oscillate. Let's break down how this oscillation happens:
### How an LC Oscillator Works:
1. **Initial Energy Storage**:
- Initially, energy is supplied to the system by charging the capacitor. This can be done by applying a voltage across the capacitor, causing it to store electric charge. At this stage, the inductor does not have any current passing through it, and all the energy is stored in the electric field of the capacitor.
2. **Energy Transfer to the Inductor**:
- Once the capacitor is charged, the circuit is closed, and the capacitor starts to discharge. As it discharges, current flows through the inductor. According to **Lenz's Law**, the inductor resists changes in current, but as current flows through it, a magnetic field builds up around it.
- At this point, the energy that was stored in the capacitor’s electric field is transferred to the inductor’s magnetic field.
3. **Inductor Generates Current**:
- As the capacitor loses its charge, its voltage drops to zero, but now the inductor's magnetic field is fully developed. When the capacitor is fully discharged, the energy stored in the inductor's magnetic field starts to induce a current in the circuit (due to **Faraday's Law of Induction**).
- This induced current starts to recharge the capacitor, but with the opposite polarity (because the direction of current has changed). As the inductor's magnetic field collapses, this energy flows back into the capacitor, but with reversed polarity.
4. **Reverse Charging of the Capacitor**:
- The energy now flows back into the capacitor, causing it to charge again, but this time with opposite polarity compared to the initial state. The cycle now repeats, with the capacitor discharging and the inductor storing energy in its magnetic field, and then the inductor inducing current back to recharge the capacitor.
5. **Oscillation Continues**:
- This back-and-forth transfer of energy between the capacitor and the inductor causes an oscillation. The frequency of this oscillation depends on the values of the inductor (L) and capacitor (C) and can be determined by the formula:
\[
f = \frac{1}{2\pi\sqrt{LC}}
\]
where:
- \( f \) is the frequency of oscillation,
- \( L \) is the inductance in henries (H),
- \( C \) is the capacitance in farads (F).
This formula shows that the frequency of the oscillation is inversely proportional to the square root of the inductance and capacitance. Higher inductance or capacitance values lead to lower oscillation frequencies, while lower values produce higher frequencies.
### Factors That Influence Oscillation:
1. **Resistance**:
- In a practical LC oscillator, resistance (from wires and components) is always present, which causes energy to be lost as heat. Over time, this will cause the oscillations to die out, a phenomenon known as **damping**.
- To counteract damping, an **amplifying device** (such as a transistor or operational amplifier) is added to the circuit to supply energy and sustain the oscillation. This ensures the circuit oscillates continuously without losing energy.
2. **Feedback**:
- A crucial part of a practical LC oscillator is **positive feedback**. The oscillator needs a portion of the output signal to be fed back into the input in phase with the original signal. This positive feedback sustains the oscillation, compensating for energy lost due to resistance.
- Feedback is often provided through a transistor or amplifier that boosts the signal and maintains the amplitude of oscillation.
### Common Types of LC Oscillators:
1. **Colpitts Oscillator**:
- In this design, a single inductor is used, and the capacitor is divided into two parts. The feedback is taken from the junction of the two capacitors, making the circuit stable and widely used in high-frequency applications.
2. **Hartley Oscillator**:
- In this design, a single capacitor is used, and the inductor is divided into two parts. The feedback is taken from the junction of the two inductors.
3. **Clapp Oscillator**:
- Similar to the Colpitts oscillator but with an additional capacitor added in series with the inductor to improve frequency stability.
4. **Crystal Oscillators**:
- Though these technically use a piezoelectric crystal rather than a traditional LC circuit, they operate on the same principle of resonance and energy transfer, providing highly stable frequencies.
### Applications of LC Oscillators:
- **Radio Transmitters**: LC oscillators are used to generate carrier waves at specific frequencies for transmitting signals.
- **Signal Generators**: These circuits are used in labs and testing equipment to generate signals for testing.
- **Clock Circuits**: In some older systems or specific applications, LC oscillators are used for timing circuits, though crystal oscillators are more common today for higher precision.
- **RF Oscillators**: LC oscillators are crucial in generating high-frequency signals in communication systems like radios, televisions, and cell phones.
### Conclusion:
An LC oscillator works by continuously transferring energy between an inductor and a capacitor in a circuit. The oscillation frequency depends on the values of the inductor and capacitor, and additional feedback circuitry is used to sustain the oscillation in practical systems. This type of oscillator is widely used in applications requiring stable and continuous wave generation at specific frequencies.
### Basic Principles
1. **Components**:
- **Inductor (L)**: Stores energy in the form of a magnetic field.
- **Capacitor (C)**: Stores energy in the form of an electric field.
2. **Oscillation Mechanism**:
- **Energy Exchange**: The LC circuit works by continuously exchanging energy between the inductor and the capacitor.
### Working of the LC Oscillator
1. **Initial State**:
- When the capacitor is charged and the switch is closed, it starts discharging through the inductor. At this moment, the capacitor is fully charged, and the current through the inductor is zero.
2. **Discharge and Oscillation**:
- **Capacitor Discharge**: As the capacitor discharges, it releases its stored energy into the inductor. The current through the inductor starts to increase, and as it does, the magnetic field builds up around the inductor.
- **Energy Transfer**: The inductor resists changes in current due to its inductance, causing the current to rise and the capacitor to discharge further.
3. **Maximum Current**:
- **Inductor's Role**: Once the capacitor is fully discharged, the inductor has its maximum current flowing through it. The energy stored in the inductor's magnetic field is now at its peak.
- **Capacitor Recharging**: The inductor’s current starts recharging the capacitor but with the opposite polarity.
4. **Capacitor Charging and Oscillation Continuation**:
- **Charging Process**: As the capacitor charges up again, the current through the inductor starts to decrease. The energy stored in the inductor’s magnetic field is transferred back to the capacitor’s electric field.
- **Oscillation**: This process continues back and forth, causing the capacitor and inductor to oscillate in terms of energy exchange. The system will oscillate at its natural resonant frequency, which is determined by the values of L and C.
### Resonant Frequency
The frequency of oscillation is given by the formula:
\[ f = \frac{1}{2 \pi \sqrt{LC}} \]
where:
- \( f \) is the resonant frequency,
- \( L \) is the inductance,
- \( C \) is the capacitance.
### Damping and Practical Considerations
- **Ideal vs. Real**: In an ideal LC circuit with no resistance, the oscillations would continue indefinitely. However, in practical circuits, resistive components and other losses cause damping, which gradually reduces the amplitude of the oscillations unless energy is supplied continuously.
- **Additional Components**: To sustain oscillations, an active component like a transistor or operational amplifier is often added to the circuit to compensate for losses and maintain oscillations.
### Summary
In essence, an LC oscillator uses the interplay between inductance and capacitance to create a resonant frequency at which it naturally oscillates. This principle is widely used in various electronic applications, such as in radio transmitters and receivers, where stable frequencies are crucial.

Applied Physics

Signals and Systems

Digital Electronics
Basic Concepts
Basic Laws
Units

Ohmic Resistors
Capacitors and Inductors

RC Circuit
First-Order Circuits
Second-Order Circuits
Principles Of Circuit Analysis
Sinusoids and Phasors
AC Steady-State Analysis
Single Phase A.C. Circuits
Three-Phase Circuits
Resonance In Series And Parallel Circuits
Network Theorems
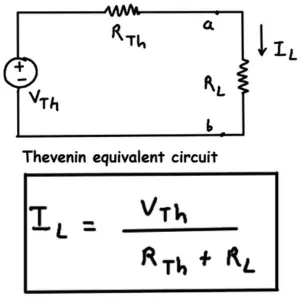
Thevenin's Theorem
Two-port Networks
Digital Electronics
Oscilloscope

Ohmmeter

Voltmeter
Ammeter

Induction Motor
Transformer
Operational Amplifiers
Components
Symbols
Formulas
EE Notes
EE Dictionary

MCQ Quiz

Interview Q&A
Power Electronics Book
Advanced Calculator

Basic Calculator
Simulator
Videos
Q&A

Capacitance Meter
Two Way Switch
Electrical Machines
Power Electronics

Electrical Drives & Their Control

Electrical Safety & Standards

Basics of Electronics Engineering
Electromagnetic Fields

Electrical Machines

More Items Coming Soon...