2 Answers
### Detailed Explanation
1. **Definition**:
- Transconductance is defined as the derivative of the drain current with respect to the gate-source voltage while keeping the drain-source voltage (\( V_{DS} \)) constant:
\[
g_m = \frac{\partial I_D}{\partial V_{GS}} \bigg|_{V_{DS} = \text{constant}}
\]
- Essentially, it represents how much the drain current (\( I_D \)) changes for a small change in the gate-source voltage (\( V_{GS} \)).
2. **Significance**:
- **Amplification**: Transconductance is crucial in determining the gain of an amplifier circuit that uses MOSFETs. Higher \( g_m \) means that a MOSFET can produce a larger change in drain current for a given change in gate voltage, which enhances the amplification capability.
- **Switching Speed**: In digital circuits, higher transconductance can contribute to faster switching speeds because the MOSFET can more quickly adjust its current in response to changes in the gate voltage.
3. **Expression for \( g_m \)**:
- For an n-channel MOSFET in the saturation region (which is where it's commonly used as an amplifier), transconductance can be approximated by:
\[
g_m = \frac{2 I_D}{V_{GS} - V_{th}}
\]
where \( V_{th} \) is the threshold voltage of the MOSFET. This shows that \( g_m \) is proportional to the drain current (\( I_D \)) and inversely proportional to the overdrive voltage (\( V_{GS} - V_{th} \)).
4. **Practical Considerations**:
- **Device Sizing**: In practical circuit design, adjusting the size of the MOSFET (such as the width-to-length ratio, \( W/L \)) can influence \( g_m \). A larger MOSFET typically has a higher \( g_m \) due to its ability to handle more current.
- **Biasing Conditions**: The operating point of the MOSFET (how it's biased) affects \( g_m \). For accurate results, measurements or calculations of \( g_m \) need to consider the specific operating conditions of the MOSFET.
5. **Importance in Circuit Design**:
- In analog circuits, such as amplifiers, \( g_m \) is a critical factor in determining the gain and linearity of the amplifier. In digital circuits, it impacts the speed and efficiency of switching operations.
In summary, transconductance is a fundamental parameter that quantifies how effectively a MOSFET translates gate voltage changes into drain current changes. It plays a vital role in both analog and digital circuit design, influencing amplification, switching speed, and overall circuit performance.
Here's a more detailed explanation:
### Definition
Transconductance \( g_{m} \) is defined as the rate of change of the drain current (\( I_{D} \)) with respect to the gate-to-source voltage (\( V_{GS} \)), while keeping the drain-to-source voltage (\( V_{DS} \)) constant. Mathematically, it is expressed as:
\[ g_{m} = \frac{\partial I_{D}}{\partial V_{GS}} \]
### Importance in MOSFETs
1. **Amplification Efficiency**: Transconductance is a measure of how effectively a MOSFET can amplify signals. A higher \( g_{m} \) means that small changes in the gate voltage result in large changes in the drain current, which is desirable for amplifying weak signals.
2. **Voltage-Controlled Current Source**: MOSFETs are often used as voltage-controlled current sources. The transconductance indicates how sensitive the drain current is to changes in gate voltage, making it crucial for designing circuits like amplifiers and analog switches.
3. **Gain of Amplifiers**: In analog circuits, particularly in amplifier design, transconductance directly impacts the gain of the amplifier. The voltage gain (\( A_v \)) of a MOSFET amplifier can be approximated as:
\[ A_v \approx g_{m} \times R_{L} \]
where \( R_{L} \) is the load resistance. Thus, higher transconductance leads to higher gain.
### Calculation
For a MOSFET in its active (or saturation) region, transconductance can be approximated as:
\[ g_{m} = \frac{2 I_{D}}{V_{GS} - V_{th}} \]
where:
- \( I_{D} \) is the drain current,
- \( V_{GS} \) is the gate-to-source voltage,
- \( V_{th} \) is the threshold voltage of the MOSFET.
Alternatively, in terms of the MOSFET’s process parameters and biasing conditions, \( g_{m} \) can also be expressed as:
\[ g_{m} = \sqrt{2 k' \cdot W/L \cdot I_{D}} \]
where:
- \( k' \) is the process transconductance parameter,
- \( W \) is the width of the MOSFET’s gate,
- \( L \) is the length of the MOSFET’s gate.
### Practical Considerations
- **Temperature Dependence**: \( g_{m} \) can vary with temperature because the threshold voltage \( V_{th} \) and mobility of carriers in the MOSFET change with temperature.
- **Device Scaling**: As MOSFETs are scaled down in size for higher density integration, \( g_{m} \) can increase because smaller devices can have higher \( I_{D} \) for the same gate voltage. However, this scaling also brings challenges like increased short-channel effects that might affect \( g_{m} \).
Understanding and optimizing transconductance is essential for designing efficient and high-performance analog and mixed-signal circuits using MOSFETs.

Applied Physics

Signals and Systems

Digital Electronics
Basic Concepts
Basic Laws
Units

Ohmic Resistors
Capacitors and Inductors

RC Circuit
First-Order Circuits
Second-Order Circuits
Principles Of Circuit Analysis
Sinusoids and Phasors
AC Steady-State Analysis
Single Phase A.C. Circuits
Three-Phase Circuits
Resonance In Series And Parallel Circuits
Network Theorems
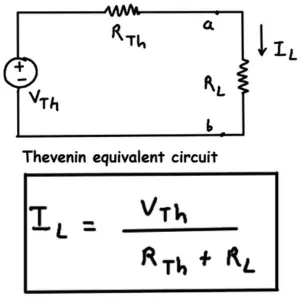
Thevenin's Theorem
Two-port Networks
Digital Electronics
Oscilloscope

Ohmmeter

Voltmeter
Ammeter

Induction Motor
Transformer
Operational Amplifiers
Components
Symbols
Formulas
EE Notes
EE Dictionary

MCQ Quiz

Interview Q&A
Power Electronics Book
Advanced Calculator

Basic Calculator
Simulator
Videos
Q&A

Capacitance Meter
Two Way Switch
Electrical Machines
Power Electronics

Electrical Drives & Their Control

Electrical Safety & Standards

Basics of Electronics Engineering
Electromagnetic Fields

Electrical Machines

More Items Coming Soon...