2 Answers
Here’s a detailed explanation of the working principle:
### 1. **Components of PID Controller**
The PID controller is made up of three distinct components, each addressing a different aspect of the control process:
#### a. Proportional (P) Control
- **Function**: The proportional component produces an output that is proportional to the current error value.
- **Formula**: \( P = K_p \times e(t) \)
- \( K_p \) is the proportional gain.
- \( e(t) \) is the error at time \( t \), which is the difference between the setpoint and the process variable.
- **Effect**: This component adjusts the control input in proportion to the current error. If the error is large, the output will be large, aiming to correct the error quickly.
#### b. Integral (I) Control
- **Function**: The integral component addresses the accumulated past errors by integrating the error over time.
- **Formula**: \( I = K_i \times \int e(t) \, dt \)
- \( K_i \) is the integral gain.
- **Effect**: This term helps eliminate residual steady-state errors that the proportional control alone might not be able to correct. It sums up the past errors and applies a correction based on this accumulated error.
#### c. Derivative (D) Control
- **Function**: The derivative component predicts future errors based on the rate of change of the error.
- **Formula**: \( D = K_d \times \frac{d e(t)}{dt} \)
- \( K_d \) is the derivative gain.
- **Effect**: This term provides a damping effect by considering how fast the error is changing. It anticipates the future behavior of the error and applies a corrective action to reduce overshoot and improve stability.
### 2. **Combined Output**
The PID controller combines these three components to produce the control output:
\[ u(t) = P + I + D \]
\[ u(t) = K_p \times e(t) + K_i \times \int e(t) \, dt + K_d \times \frac{d e(t)}{dt} \]
### 3. **Operation**
- **Error Calculation**: The controller continuously measures the process variable (PV) and calculates the error \( e(t) = \text{Setpoint} - \text{Process Variable} \).
- **Control Action**: Based on the calculated error, the PID controller adjusts the control input to the process, aiming to bring the process variable closer to the setpoint.
- **Tuning**: The performance of the PID controller depends on the values of \( K_p \), \( K_i \), and \( K_d \). These parameters are adjusted through a process called tuning to achieve the desired response characteristics, such as fast response time, minimal overshoot, and stable operation.
### 4. **Applications**
PID controllers are used in various applications, including:
- **Temperature Control**: To maintain a desired temperature in heating systems.
- **Speed Control**: For controlling the speed of motors.
- **Position Control**: In systems like robotics and automation where precise positioning is required.
- **Level Control**: To maintain the level of a fluid in tanks.
### 5. **Advantages and Limitations**
**Advantages:**
- **Simplicity**: PID controllers are straightforward to implement and understand.
- **Versatility**: They can be applied to a wide range of systems and processes.
- **Effectiveness**: They can handle a variety of dynamic behaviors and achieve desired performance.
**Limitations:**
- **Tuning Complexity**: Finding the optimal parameters \( K_p \), \( K_i \), and \( K_d \) can be challenging and may require trial and error.
- **Noise Sensitivity**: The derivative component can amplify noise in the error signal, potentially causing instability.
In summary, the PID controller uses proportional, integral, and derivative actions to continuously adjust the control input and minimize the error between the setpoint and the process variable. Proper tuning of the controller parameters is essential for achieving the best performance.
### Working Principle of PID Controller
The PID controller works based on three key components:
1. **Proportional (P) Control:**
- The proportional part of the controller generates an output that is directly proportional to the current error value.
- **Error (e) = Setpoint (SP) - Process Variable (PV)**
- The proportional response is calculated as:
**P_out = Kp * e**
- Here, `Kp` is the proportional gain. If the gain is too high, the system can become unstable, while if it’s too low, the response might be too slow.
2. **Integral (I) Control:**
- The integral component sums up the past errors over time and multiplies it by the integral gain. This helps eliminate the residual steady-state error that occurs when only using proportional control.
- The integral response is calculated as:
**I_out = Ki * ∫e(t) dt**
- Here, `Ki` is the integral gain, and `∫e(t) dt` represents the integral of the error over time. The integral action increases over time if the error persists, pushing the system towards the setpoint.
3. **Derivative (D) Control:**
- The derivative component predicts the future error based on its rate of change and applies a corrective action accordingly. This helps to dampen the oscillations and improve system stability.
- The derivative response is calculated as:
**D_out = Kd * de(t)/dt**
- Here, `Kd` is the derivative gain, and `de(t)/dt` represents the rate of change of the error. If the error is changing rapidly, the derivative term provides a significant contribution to counteract this change.
### Combined PID Control Output
The overall output of the PID controller is the sum of these three components:
**PID_out = P_out + I_out + D_out**
Or in terms of the gains and error:
**PID_out = Kp * e + Ki * ∫e(t) dt + Kd * de(t)/dt**
### How PID Works in a System
1. **Proportional Control:** As soon as the system starts deviating from the setpoint, the proportional term kicks in and applies a correction that is proportional to the error. However, this alone might not bring the error to zero due to a residual steady-state error.
2. **Integral Control:** The integral term starts accumulating the error over time and adds it to the output. This helps to reduce any steady-state error that the proportional control cannot eliminate.
3. **Derivative Control:** The derivative term looks at how quickly the error is changing and applies a damping effect, which helps in reducing overshoot and settling time, leading to a more stable system response.
### Tuning a PID Controller
- **Tuning** refers to adjusting the `Kp`, `Ki`, and `Kd` gains to achieve the desired system performance. Poorly tuned controllers can lead to instability, slow response, or excessive oscillations. Common methods for tuning include trial and error, Ziegler-Nichols method, and software-based tuning.
### Applications of PID Controllers
PID controllers are widely used in various applications such as:
- Temperature control systems
- Speed control in motors
- Process control in chemical industries
- Robotics
- Automatic voltage regulators (AVRs)
The versatility and effectiveness of PID controllers make them a popular choice in industries where precise control is essential.

Applied Physics

Signals and Systems

Digital Electronics
Basic Concepts
Basic Laws
Units

Ohmic Resistors
Capacitors and Inductors

RC Circuit
First-Order Circuits
Second-Order Circuits
Principles Of Circuit Analysis
Sinusoids and Phasors
AC Steady-State Analysis
Single Phase A.C. Circuits
Three-Phase Circuits
Resonance In Series And Parallel Circuits
Network Theorems
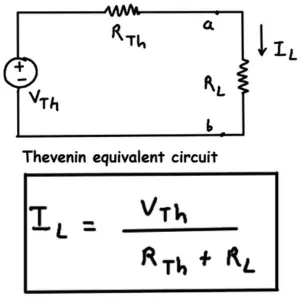
Thevenin's Theorem
Two-port Networks
Digital Electronics
Oscilloscope

Ohmmeter

Voltmeter
Ammeter

Induction Motor
Transformer
Operational Amplifiers
Components
Symbols
Formulas
EE Notes
EE Dictionary

MCQ Quiz

Interview Q&A
Power Electronics Book
Advanced Calculator

Basic Calculator
Simulator
Videos
Q&A

Capacitance Meter
Two Way Switch
Electrical Machines
Power Electronics

Electrical Drives & Their Control

Electrical Safety & Standards

Basics of Electronics Engineering
Electromagnetic Fields

Electrical Machines

More Items Coming Soon...