1 Answer
### Key Concept:
In classical physics, particles like electrons or baseballs are seen as discrete objects with well-defined positions and velocities. However, quantum mechanics introduced the idea that on very small scales (atomic or subatomic), particles exhibit both particle-like and wave-like properties, a phenomenon known as **wave-particle duality**.
According to de Broglie’s hypothesis, just like light (which can behave both as a wave and as a particle), particles could also behave as waves. The **de Broglie wavelength** is the wavelength associated with a moving particle and is inversely proportional to its momentum.
### Formula:
The de Broglie wavelength \( \lambda \) is given by the equation:
\[
\lambda = \frac{h}{p}
\]
Where:
- \( \lambda \) is the de Broglie wavelength.
- \( h \) is **Planck's constant** (\(6.626 \times 10^{-34} \, \text{J} \cdot \text{s}\)).
- \( p \) is the **momentum** of the particle, which is the product of its mass \( m \) and velocity \( v \) (\( p = mv \)).
Thus, the de Broglie wavelength is inversely related to the momentum of the particle: the faster the particle moves or the more massive it is, the shorter its wavelength. Conversely, the slower the particle moves or the less massive it is, the longer its wavelength.
### Wave-Particle Duality:
To better understand the wave-like nature of particles, think about light, which can also act as both a particle (photon) and a wave. Light waves have a wavelength, and de Broglie suggested that this could apply to particles as well, although the wavelength for everyday objects would be so incredibly small that it would be unnoticeable.
For example:
- For very small particles like electrons, the de Broglie wavelength can be significant enough to observe in experiments (such as electron diffraction).
- For large objects like a baseball, the wavelength becomes so tiny that it is practically undetectable. A baseball with a mass of around 0.145 kg moving at 20 m/s would have an extraordinarily small de Broglie wavelength, far too small to be observed with current technology.
### Importance of the De Broglie Wavelength:
The concept of the de Broglie wavelength led to several significant developments in physics, especially in quantum mechanics:
1. **Electron Diffraction**: One of the most notable consequences of the de Broglie wavelength is that electrons (and other particles) can be diffracted just like light waves. This was experimentally verified in 1927 when electrons were shown to create diffraction patterns, confirming their wave-like nature.
2. **Heisenberg's Uncertainty Principle**: The wave-particle duality introduced by de Broglie laid the foundation for other important quantum concepts, like Heisenberg's uncertainty principle, which deals with the limits of simultaneously measuring a particle’s position and momentum.
3. **Quantum Mechanics and Atomic Structure**: The idea that electrons can behave as waves was critical in developing the quantum mechanical model of the atom. It helped explain the discrete energy levels observed in atoms, as only certain wavelengths of electron waves would fit in the confined spaces around the nucleus.
### Practical Example:
Let’s take an example of a moving electron:
1. An electron with a velocity of \(v = 1 \times 10^6 \, \text{m/s}\) and mass \(m = 9.11 \times 10^{-31} \, \text{kg}\).
2. The momentum of the electron is \(p = mv = 9.11 \times 10^{-31} \times 1 \times 10^6 = 9.11 \times 10^{-25} \, \text{kg} \cdot \text{m/s}\).
3. Using de Broglie’s formula, the wavelength is:
\[
\lambda = \frac{6.626 \times 10^{-34}}{9.11 \times 10^{-25}} = 7.27 \times 10^{-10} \, \text{m}
\]
This wavelength is in the range of X-rays, which is significant for phenomena like electron diffraction in physics experiments.
### Summary:
The de Broglie wavelength shows that every particle, no matter how large, has an associated wavelength that depends on its momentum. The wave-like nature of particles becomes evident at microscopic scales, leading to the wave-particle duality that is fundamental in quantum mechanics. The de Broglie hypothesis not only explained the wave behavior of particles but also influenced the development of quantum theory, making it one of the cornerstone ideas in understanding the behavior of particles at the atomic and subatomic levels.

Applied Physics

Signals and Systems

Digital Electronics
Basic Concepts
Basic Laws
Units

Ohmic Resistors
Capacitors and Inductors

RC Circuit
First-Order Circuits
Second-Order Circuits
Principles Of Circuit Analysis
Sinusoids and Phasors
AC Steady-State Analysis
Single Phase A.C. Circuits
Three-Phase Circuits
Resonance In Series And Parallel Circuits
Network Theorems
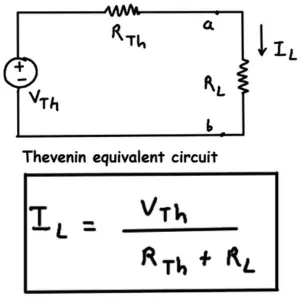
Thevenin's Theorem
Two-port Networks
Digital Electronics
Oscilloscope

Ohmmeter

Voltmeter
Ammeter

Induction Motor
Transformer
Operational Amplifiers
Components
Symbols
Formulas
EE Notes
EE Dictionary

MCQ Quiz

Interview Q&A
Power Electronics Book
Advanced Calculator

Basic Calculator
Simulator
Videos
Q&A

Capacitance Meter
Two Way Switch
Electrical Machines
Power Electronics

Electrical Drives & Their Control

Electrical Safety & Standards

Basics of Electronics Engineering
Electromagnetic Fields

Electrical Machines

More Items Coming Soon...