2 Answers
### 1. **For Synchronous Motors**
The speed of a synchronous motor is determined by the frequency of the electrical supply and the number of poles of the motor. Synchronous motors run at a constant speed, which is known as the **synchronous speed**.
#### Formula:
\[
N_s = \frac{120 \times f}{P}
\]
- **\(N_s\)** = Synchronous speed (in revolutions per minute, or RPM)
- **\(f\)** = Frequency of the electrical supply (in Hertz, or Hz)
- **\(P\)** = Number of poles in the motor
#### Example:
If you have a motor with 4 poles and it is connected to a 50 Hz power supply, the synchronous speed would be calculated as:
\[
N_s = \frac{120 \times 50}{4} = 1500 \, \text{RPM}
\]
### 2. **For Induction Motors**
Induction motors do not run at synchronous speed. They always run at a slightly lower speed due to something called **slip**. Slip accounts for the difference between the synchronous speed and the actual rotor speed. The actual rotor speed is given by:
\[
N_r = N_s \times (1 - S)
\]
Where:
- **\(N_r\)** = Rotor speed (in RPM)
- **\(N_s\)** = Synchronous speed (in RPM, calculated with the same formula as above)
- **\(S\)** = Slip (a fraction, typically between 0 and 1)
#### Slip Calculation:
The slip \(S\) is given by:
\[
S = \frac{N_s - N_r}{N_s}
\]
Rearranging this formula to calculate the rotor speed \(N_r\), we get:
\[
N_r = N_s \times (1 - S)
\]
#### Example:
Let’s say you have an induction motor with a synchronous speed of 1500 RPM (as calculated before), and the slip is 4% (or 0.04 in decimal form). The actual rotor speed can be calculated as:
\[
N_r = 1500 \times (1 - 0.04) = 1500 \times 0.96 = 1440 \, \text{RPM}
\]
### Important Notes:
1. **Slip (S)** is necessary for torque generation in induction motors. Without slip, no relative motion between the rotor and the stator magnetic field would exist, and no torque would be generated.
2. In **synchronous motors**, the rotor locks into the rotating magnetic field and runs exactly at synchronous speed, so there is no slip.
3. **Poles (P)**: The number of poles is determined by the motor’s construction, and higher pole numbers result in slower motor speeds.
### Additional Variables for Specific Calculations:
- **Rotor Frequency**: In an induction motor, the rotor frequency (the frequency at which the rotor’s magnetic field rotates) can be calculated by:
\[
f_r = S \times f
\]
Where **\(f_r\)** is the rotor frequency, and **\(f\)** is the supply frequency.
### Steps to Calculate Rotor Speed:
1. Determine the number of poles **\(P\)** and the supply frequency **\(f\)** of the motor.
2. Use the formula to find the synchronous speed **\(N_s\)**.
3. If dealing with an induction motor, calculate the slip **\(S\)** or find it in the motor's specifications.
4. Calculate the rotor speed **\(N_r\)** using the slip and synchronous speed.
### Summary:
- **Synchronous motor**: Rotor speed equals the synchronous speed, calculated using \(N_s = \frac{120 \times f}{P}\).
- **Induction motor**: Rotor speed is lower than synchronous speed, calculated using \(N_r = N_s \times (1 - S)\), where \(S\) is the slip.
### 1. **Synchronous Speed (Ns)**
Synchronous speed is the theoretical speed at which the magnetic field rotates in the stator, and it's given by the following formula:
\[
N_s = \frac{120 \cdot f}{P}
\]
- \(N_s\) = Synchronous speed (in RPM)
- \(f\) = Supply frequency (in Hz)
- \(P\) = Number of poles in the motor
For example, if the supply frequency is 50 Hz and the motor has 4 poles:
\[
N_s = \frac{120 \cdot 50}{4} = 1500 \, \text{RPM}
\]
### 2. **Rotor Speed (Nr)**
Rotor speed is the actual speed at which the rotor spins. Due to **slip**, the rotor speed is slightly less than the synchronous speed in an induction motor. The slip speed is a percentage of the synchronous speed, and the rotor speed can be calculated as:
\[
N_r = N_s \cdot (1 - s)
\]
Where:
- \(N_r\) = Rotor speed (in RPM)
- \(s\) = Slip, a ratio of the difference between synchronous speed and rotor speed to synchronous speed. It’s typically expressed as a percentage and can be calculated as:
\[
s = \frac{N_s - N_r}{N_s}
\]
### 3. **Calculating Slip**
The slip can be calculated as a percentage of the synchronous speed:
\[
s = \frac{N_s - N_r}{N_s} \times 100
\]
Or, if the motor's slip is known in percentage form, you can calculate the rotor speed directly:
\[
N_r = N_s \cdot (1 - \frac{s}{100})
\]
### Example:
Let’s assume:
- Supply frequency \( f = 50 \, \text{Hz} \)
- Number of poles \( P = 4 \)
- Slip \( s = 5\% \)
1. **Find the synchronous speed**:
\[
N_s = \frac{120 \cdot 50}{4} = 1500 \, \text{RPM}
\]
2. **Calculate the rotor speed** using the slip value:
\[
N_r = 1500 \cdot (1 - \frac{5}{100}) = 1500 \cdot 0.95 = 1425 \, \text{RPM}
\]
Thus, the rotor speed is 1425 RPM.
### Key Notes:
- **Synchronous speed** depends only on the supply frequency and the number of poles in the motor.
- **Rotor speed** is always less than the synchronous speed due to slip in induction motors.
- Slip varies with load; a higher load generally increases slip, which reduces rotor speed.
Would you like to calculate the rotor speed for a specific motor, or dive deeper into the concept of slip and how it affects performance?

Applied Physics

Signals and Systems

Digital Electronics
Basic Concepts
Basic Laws
Units

Ohmic Resistors
Capacitors and Inductors

RC Circuit
First-Order Circuits
Second-Order Circuits
Principles Of Circuit Analysis
Sinusoids and Phasors
AC Steady-State Analysis
Single Phase A.C. Circuits
Three-Phase Circuits
Resonance In Series And Parallel Circuits
Network Theorems
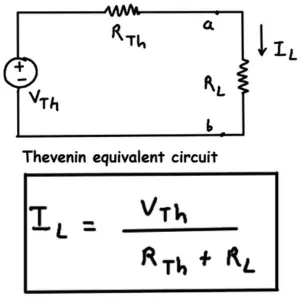
Thevenin's Theorem
Two-port Networks
Digital Electronics
Oscilloscope

Ohmmeter

Voltmeter
Ammeter

Induction Motor
Transformer
Operational Amplifiers
Components
Symbols
Formulas
EE Notes
EE Dictionary

MCQ Quiz

Interview Q&A
Power Electronics Book
Advanced Calculator

Basic Calculator
Simulator
Videos
Q&A

Capacitance Meter
Two Way Switch
Electrical Machines
Power Electronics

Electrical Drives & Their Control

Electrical Safety & Standards

Basics of Electronics Engineering
Electromagnetic Fields

Electrical Machines

More Items Coming Soon...